Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Wang, Weike
and
Yang, Tong
2007.
Existence and stability of planar diffusion waves for 2-D Euler equations with damping.
Journal of Differential Equations,
Vol. 242,
Issue. 1,
p.
40.
Yang, Xiongfeng
and
Wang, Weike
2007.
The suppressible property of the solution for three-dimensional Euler equations with damping.
Nonlinear Analysis: Real World Applications,
Vol. 8,
Issue. 1,
p.
53.
Said-Houari, Belkacem
2009.
Convergence to strong nonlinear diffusion waves for solutions to p-system with damping.
Journal of Differential Equations,
Vol. 247,
Issue. 3,
p.
917.
Mei, Ming
2009.
Nonlinear diffusion waves for hyperbolic p-system with nonlinear damping.
Journal of Differential Equations,
Vol. 247,
Issue. 4,
p.
1275.
Liao, Jie
Wang, Weike
and
Yang, Tong
2009.
Lp convergence rates of planar waves for multi-dimensional Euler equations with damping.
Journal of Differential Equations,
Vol. 247,
Issue. 1,
p.
303.
Ma, Hongfang
and
Mei, Ming
2010.
Best asymptotic profile for linear damped p-system with boundary effect.
Journal of Differential Equations,
Vol. 249,
Issue. 2,
p.
446.
Geng, Shifeng
and
Wang, Zhen
2010.
Convergence Rates to Nonlinear Diffusion Waves for Solutions to the System of Compressible Adiabatic Flow through Porous Media.
Communications in Partial Differential Equations,
Vol. 36,
Issue. 5,
p.
850.
Ma, Hongfang
2010.
Optimal convergence rates to nonlinear diffusion waves for the compressible Euler–Poisson system with damping.
Journal of Mathematical Analysis and Applications,
Vol. 370,
Issue. 2,
p.
511.
Mei, Ming
2010.
Best Asymptotic Profile for Hyperbolicp-System with Damping.
SIAM Journal on Mathematical Analysis,
Vol. 42,
Issue. 1,
p.
1.
Huang, Feimin
Mei, Ming
and
Wang, Yong
2011.
Large Time Behavior of Solutions ton-Dimensional Bipolar Hydrodynamic Models for Semiconductors.
SIAM Journal on Mathematical Analysis,
Vol. 43,
Issue. 4,
p.
1595.
Geng, Shifeng
and
Wang, Zhen
2012.
Best asymptotic profile for the system of compressible adiabatic flow through porous media on quadrant.
Communications on Pure & Applied Analysis,
Vol. 11,
Issue. 2,
p.
475.
Huang, Feimin
Mei, Ming
Wang, Yong
and
Yang, Tong
2012.
Long-time Behavior of Solutions to the Bipolar Hydrodynamic Model of Semiconductors with Boundary Effect.
SIAM Journal on Mathematical Analysis,
Vol. 44,
Issue. 2,
p.
1134.
Geng, Shifeng
and
Wang, Zhen
2014.
Lpconvergence rates of solutions for n -dimensional quasilinear damped wave equation.
Journal of Mathematical Analysis and Applications,
Vol. 412,
Issue. 1,
p.
449.
Geng, Shifeng
and
Zhang, Lina
2014.
Large-time behavior of solutions for the system of compressible adiabatic flow through porous media with nonlinear damping.
Communications on Pure and Applied Analysis,
Vol. 13,
Issue. 6,
p.
2211.
Li, Haitong
Li, Jingyu
Mei, Ming
and
Zhang, Kaijun
2017.
Convergence to nonlinear diffusion waves for solutions of p-system with time-dependent damping.
Journal of Mathematical Analysis and Applications,
Vol. 456,
Issue. 2,
p.
849.
LI, Xing
and
YONG, Yan
2017.
Large time behavior of solutions to 1-dimensional bipolar quantum hydrodynamic model for semiconductors.
Acta Mathematica Scientia,
Vol. 37,
Issue. 3,
p.
806.
Li, Haitong
Li, Jingyu
Mei, Ming
and
Zhang, Kaijun
2019.
Asymptotic behavior of solutions to bipolar Euler–Poisson equations with time-dependent damping.
Journal of Mathematical Analysis and Applications,
Vol. 473,
Issue. 2,
p.
1081.
Xu, Dongsheng
and
Yang, Xiongfeng
2021.
The asymptotic profile of solutions to damped Euler equations.
ZAMM - Journal of Applied Mathematics and Mechanics / Zeitschrift für Angewandte Mathematik und Mechanik,
Vol. 101,
Issue. 10,
Geng, Shifeng
Huang, Feimin
and
Wu, Xiaochun
2022.
L2-convergence to nonlinear diffusion waves for Euler equations with time-dependent damping.
Acta Mathematica Scientia,
Vol. 42,
Issue. 6,
p.
2505.
Zhang, Nangao
2022.
Optimal convergence rates to diffusion waves for solutions of p-system with damping on quadrant.
Journal of Mathematical Analysis and Applications,
Vol. 512,
Issue. 1,
p.
126118.
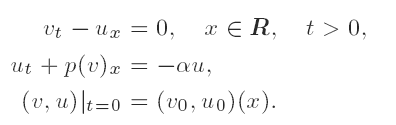 Hsiao and Liu showed that the solution (υ, u) behaves as the diffusion wave (ῡ, ū), i.e. the solution of the porous-media equation due to the Darcy law. The optimal convergence rates have been obtained by Nishihara and co-workers. When υ0(x) has the same constant state at x = ±∞, the convergence rate ‖(υ − ῡ)(·,t)‖L∞ = O(t−1) obtained is ‘optimal’, since ‖ῡ(·,t)‖∞ = O(t−1/2). However, this ‘optimal’ convergence rate is less sufficient to determine the location of the diffusion wave. Our aim in this paper is to obtain the ‘truly optimal’ convergence rate by choosing suitably located diffusion waves.
Hsiao and Liu showed that the solution (υ, u) behaves as the diffusion wave (ῡ, ū), i.e. the solution of the porous-media equation due to the Darcy law. The optimal convergence rates have been obtained by Nishihara and co-workers. When υ0(x) has the same constant state at x = ±∞, the convergence rate ‖(υ − ῡ)(·,t)‖L∞ = O(t−1) obtained is ‘optimal’, since ‖ῡ(·,t)‖∞ = O(t−1/2). However, this ‘optimal’ convergence rate is less sufficient to determine the location of the diffusion wave. Our aim in this paper is to obtain the ‘truly optimal’ convergence rate by choosing suitably located diffusion waves.