Published online by Cambridge University Press: 09 March 2021
For each recollement of triangulated categories, there is an epivalence between the middle category and the comma category associated with a triangle functor from the category on the right to the category on the left. For a morphic enhancement of a triangulated category 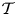 $\mathcal {T}$, there are three explicit ideals of the enhancing category, whose corresponding factor categories are all equivalent to the module category over
$\mathcal {T}$, there are three explicit ideals of the enhancing category, whose corresponding factor categories are all equivalent to the module category over 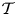 $\mathcal {T}$. Examples related to inflation categories and weighted projective lines are discussed.
$\mathcal {T}$. Examples related to inflation categories and weighted projective lines are discussed.