No CrossRef data available.
Article contents
Radial solutions of a semilinear elliptic problem*
Published online by Cambridge University Press: 14 November 2011
Synopsis
We analyse the set of nonnegative, global, and radial solutions (radial solutions, for short) of the equation
where 0 < p < 1, and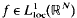 
is a radial and almost everywhere nonnegative function. We show that radial solutions of (E) exist if f(r) = o(r2p/1−1−p) or if f(r) ≈ cr2p/1−p as r → ∞, where
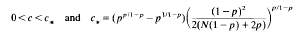
When f(r) = c*r2p/1−p + h(r) with h(r) = o(r2p/1−p) as r → ∞, radial solutions continue to exist if h(r) is sufficiently small at infinity. Existence, however, breaks down if h(r) > 0,
Whenever they exist, radial solutions are characterised in terms of their asymptotic behaviour as r → ∞.
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 118 , Issue 3-4 , 1991 , pp. 305 - 326
- Copyright
- Copyright © Royal Society of Edinburgh 1991
References
2Brezis, H.. Semilinear equations in ℝN without conditions at infinity. Appl. Math. Optim. 12 (1984), 271–282.CrossRefGoogle Scholar
3Gallouët, T. and Morel, J. M.. The equation −Δu + ∥u∥α™1 u = f for 0≦α≦1. J. Nonlinear Anal. 11 (1987), 893–912.CrossRefGoogle Scholar
4Herrero, M. A. and Velázquez, J. J. L.. On the dynamics of a semilinear heat equation with strong absorption. Comm. Partial Differential Equations 14 (1989), 1653–1715.CrossRefGoogle Scholar
5Murata, M.. Structure of positive solutions to (−Δ + V)u = 0 in ℝN. Duke Math J. 53 (1986), 869–943.CrossRefGoogle Scholar