Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Boscaggin, Alberto
Colasuonno, Francesca
and
Noris, Benedetta
2018.
Multiple positive solutions for a class of p-Laplacian Neumann problems without growth conditions.
ESAIM: Control, Optimisation and Calculus of Variations,
Vol. 24,
Issue. 4,
p.
1625.
Boscaggin, Alberto
Colasuonno, Francesca
and
De Coster, Colette
2021.
Multiple bounded variation solutions for a prescribed mean curvature equation with Neumann boundary conditions.
Journal of Differential Equations,
Vol. 285,
Issue. ,
p.
607.
Pei, Minghe
Wang, Libo
and
Lv, Xuezhe
2022.
Radial solutions for p‐Laplacian Neumann problems with gradient dependence.
Mathematische Nachrichten,
Vol. 295,
Issue. 12,
p.
2422.
Cinti, Eleonora
and
Colasuonno, Francesca
2023.
Existence and non-existence results for a semilinear fractional Neumann problem.
Nonlinear Differential Equations and Applications NoDEA,
Vol. 30,
Issue. 6,
Colasuonno, Francesca
and
Noris, Benedetta
2023.
Asymptotics for a high-energy solution of a supercritical problem.
Nonlinear Analysis,
Vol. 227,
Issue. ,
p.
113166.
Ma, Ruyun
Yang, Lijuan
and
Zhang, Yali
2024.
Global structure of positive solutions for p-Laplacian Neumann problem with indefinite weight.
Computational and Applied Mathematics,
Vol. 43,
Issue. 3,
Boscaggin, Alberto
Colasuonno, Francesca
Noris, Benedetta
and
Weth, Tobias
2024.
Multiplicity and symmetry breaking for supercritical elliptic problems in exterior domains.
Nonlinearity,
Vol. 37,
Issue. 10,
p.
105012.
Colasuonno, Francesca
Noris, Benedetta
and
Sovrano, Elisa
2025.
Continuous dependence for <inline-formula><tex-math id="M1">$ p $</tex-math></inline-formula>-Laplace equations with varying operators.
Discrete and Continuous Dynamical Systems - S,
Vol. 18,
Issue. 6,
p.
1561.
Ma, Ruyun
and
Yang, Lijuan
2025.
Global structure of positive solutions for a singular quasilinear elliptic problem.
Complex Variables and Elliptic Equations,
Vol. 70,
Issue. 2,
p.
199.
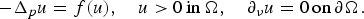 $-\Delta _pu = f(u),\quad u > 0\,{\rm in }\,\Omega ,\quad \partial _\nu u = 0\,{\rm on }\,\partial \Omega .$
$-\Delta _pu = f(u),\quad u > 0\,{\rm in }\,\Omega ,\quad \partial _\nu u = 0\,{\rm on }\,\partial \Omega .$