Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kodama, Shun
2017.
A concentration phenomenon of the least energy solution to non-autonomous elliptic problems with a totally degenerate potential.
Communications on Pure and Applied Analysis,
Vol. 16,
Issue. 2,
p.
671.


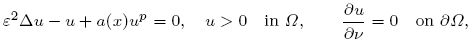
 . We show that, given ε small enough, there exists a
. We show that, given ε small enough, there exists a 