Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-26T01:17:47.071Z Has data issue: false hasContentIssue false
  • English
  • Français

On the fractional Lazer-McKenna conjecture with critical growth

Part of: Partial differential equations Elliptic equations and systems

Published online by Cambridge University Press:  11 August 2021

Qi Li  and
Shuangjie Peng
Qi Li
Affiliation:
College of Science, Wuhan University of Science and Technology, Wuhan 430065, People's Republic of China School of Mathematics and Statistics, Central China Normal University, Wuhan 430079, People's Republic of China ([email protected])
Shuangjie Peng
Affiliation:
School of Mathematics and Statistics & Hubei Key Laboratory of Mathematical Sciences, Central China Normal University, Wuhan 430079, People's Republic of China ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper deals with the following fractional elliptic equation with critical exponent

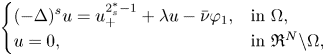\[ \begin{cases} \displaystyle (-\Delta )^{s}u=u_{+}^{2_{s}^{*}-1}+\lambda u-\bar{\nu}\varphi_{1}, & \text{in}\ \Omega,\\ \displaystyle u=0, & \text{in}\ {{\mathfrak R}}^{N}\backslash \Omega, \end{cases}\]
where $\lambda$, 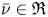$\bar {\nu }\in {{\mathfrak R}}$, 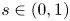$s\in (0,1)$, 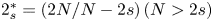$2^{*}_{s}=({2N}/{N-2s})\,(N>2s)$, $(-\Delta )^{s}$ is the fractional Laplace operator, 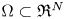$\Omega \subset {{\mathfrak R}}^{N}$ is a bounded domain with smooth boundary and $\varphi _{1}$ is the first positive eigenfunction of the fractional Laplace under the condition $u=0$ in 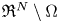${{\mathfrak R}}^{N}\setminus \Omega$. Under suitable conditions on $\lambda$ and 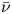$\bar {\nu }$ and using a Lyapunov-Schmidt reduction method, we prove the fractional version of the Lazer-McKenna conjecture which says that the equation above has infinitely many solutions as 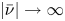$|\bar \nu | \to \infty$ .

Keywords

fractional Laplacefirst eigenfunctionLyapunov-Schmidt reduction

MSC classification

Primary: 35A01: Existence problems
Secondary: 35J60: Nonlinear elliptic equations
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 152 , Issue 4 , August 2022 , pp. 879 - 911
Check for updates
Copyright
Copyright © The Author(s), 2021. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abdellaoui, B., Dieb, A. and Mahmoudi, F.. On the fractional Lazer-McKenna conjecture with superlinear potential. Calc. Var. Partial Differ. Equ. 58 (2019), 36 pp.CrossRefGoogle Scholar
Ambrosetti, A. and Prodi, G.. On the inversion of some differentiable mappings with singularities between Banach spaces. Ann. Mat. Pura. Appl. 93 (1972), 231–246.CrossRefGoogle Scholar
Breuer, B., McKenna, P. J. and Plum, M.. Multiple solutions for a semilinear boundary value problem: a computational multiplicity proof. J. Differ. Equ. 195 (2003), 243–269.CrossRefGoogle Scholar
Caffarelli, L. and Silvestre, L.. An extension problem related to the fractional Laplacian. Comm. Partial Differ. Equ. 32 (2007), 1245–1260.CrossRefGoogle Scholar
Dancer, E. N. and Yan, S.. On the superlinear Lazer-McKenna conjecture. J. Differ. Equ. 210 (2005), 317–351.CrossRefGoogle Scholar
Dancer, E. N. and Yan, S.. On the superlinear Lazer-McKenna conjecture. II. Comm. Partial Differ. Equ. 30 (2005), 1331–1358.CrossRefGoogle Scholar
Dancer, E. N. and Yan, S.. The Lazer-McKenna conjecture and a free boundary problem in two dimensions. J. Lond. Math. Soc. 78 (2008), 639–662.CrossRefGoogle Scholar
Dávila, J., del Pino, M. and Sire, Y.. Nondegeneracy of the bubble in the critical case for nonlocal equations. Proc. Amer. Math. Soc. 141 (2013), 3865–3870.CrossRefGoogle Scholar
Dávila, J., López Rios, L. and Sire, Y.. Bubbling solutions for nonlocal elliptic problems. Rev. Mat. Iberoam 33 (2017), 509–546.CrossRefGoogle Scholar
Lazer, A. C. and McKenna, P. J.. On a conjecture related to the number of solutions of a nonlinear Dirichlet problem. Proc. Roy. Soc. Edinburgh Sect. A. 95 (1983), 275–283.CrossRefGoogle Scholar
Li, G., Yan, S. and Yang, J.. The Lazer-McKenna conjecture for an elliptic problem with critical growth. II. J. Differ. Equ. 227 (2006), 301–332.CrossRefGoogle Scholar
Li, G., Yan, S. and Yang, J.. The Lazer-McKenna conjecture for an elliptic problem with critical growth. Calc. Var. Partial Differ. Equ. 28 (2007), 471–508.CrossRefGoogle Scholar
Long, W., Yan, S. and Yang, J.. A critical elliptic problem involving fractional Laplacian operator in domains with shrinking holes. J. Differ. Equ. 267 (2019), 4117–4147.CrossRefGoogle Scholar
Nezza, E. D., Palatucci, G. and Valdinoci, E.. Hitchhiker's guide to the fractional Sobolev spaces. Bull. Sci. Math. 136 (2012), 521–573.CrossRefGoogle Scholar
Peng, S. and Wang, Z.-Q.. Segregated and synchronized vector solutions for nonlinear Schrödinger systems. Arch. Ration. Mech. Anal. 208 (2013), 305–339.CrossRefGoogle Scholar
Peng, S., Wang, Q. and Wang, Z.-Q.. On coupled nonlinear Schrödinger systems with mixed couplings. Trans. Amer. Math. Soc. 371 (2019), 7559–7583.CrossRefGoogle Scholar
Peng, S., Wang, C. and Wei, S.. Constructing solutions for the prescribed scalar curvature problem via local Pohozaev identities. J. Differ. Equ. 267 (2019), 2503–2530.CrossRefGoogle Scholar
Peng, S., Wang, C. and Yan, S.. Construction of solutions via local Pohozaev identities. J. Funct. Anal. 274 (2018), 2606–2633.CrossRefGoogle Scholar
Rey, O.. The role of the Green's function in a nonlinear elliptic equation involving the critical Sobolev exponent. J. Funct. Anal. 89 (1990), 1–52.CrossRefGoogle Scholar
Servadei, R. and Valdinoci, E.. Variational methods for non-local operators of elliptic type. Discrete Contin. Dyn. Syst. 33 (2013), 2105–2137.CrossRefGoogle Scholar
Servadei, R. and Valdinoci, E.. The Brezis-Nirenberg result for the fractional Laplacian. Trans. Amer. Math. Soc. 367 (2015), 67–102.CrossRefGoogle Scholar
Wei, J. and Yan, S.. Lazer-McKenna conjecture: the critical case. J. Funct. Anal. 244 (2007), 639–667.CrossRefGoogle Scholar
Wei, J. and Yan, S.. Infinitely many solutions for the prescribed scalar curvature problem on ${{\mathfrak R}}^{N}$. J. Funct. Anal. 258 (2010), 3048–3081.CrossRefGoogle Scholar
Wei, J. and Yan, S.. Infinitely many positive solutions for the nonlinear Schrödinger equations in ${{\mathfrak R}}^{N}$. Calc. Var. Partial Differ. Equ. 37 (2010), 423–439.CrossRefGoogle Scholar