No CrossRef data available.
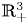 $\mathbb {R}_{+}^{3}$
$\mathbb {R}_{+}^{3}$Published online by Cambridge University Press: 07 September 2021
In this study, we continue our study of the Cauchy problem associated with the Brinkman equations [see (1.1) and (1.2) below] which model fluid flow in certain types of porous media. Here, we will consider the flow in the upper half-space
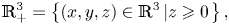 \[ \mathbb{R}_{+}^{3}=\left\{\left(x,y,z\right) \in\mathbb{R}^{3}\left\vert z\geqslant 0\right.\right\}, \]
\[ \mathbb{R}_{+}^{3}=\left\{\left(x,y,z\right) \in\mathbb{R}^{3}\left\vert z\geqslant 0\right.\right\}, \] $z=0$ is impenetrable to the fluid. This means that we will have to introduce boundary conditions that must be attached to the Brinkman equations. We study local and global well-posedness in appropriate Sobolev spaces introduced below, using Kato's theory for quasilinear equations, parabolic regularization and a comparison principle for the solutions of the problem.
$z=0$ is impenetrable to the fluid. This means that we will have to introduce boundary conditions that must be attached to the Brinkman equations. We study local and global well-posedness in appropriate Sobolev spaces introduced below, using Kato's theory for quasilinear equations, parabolic regularization and a comparison principle for the solutions of the problem.