Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Wang, Gengsheng
2002.
Optimal control problems governed by non-well-posed semilinear elliptic equation.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 49,
Issue. 3,
p.
315.
Takahashi, Futoshi
2003.
Multiple solutions of inhomogeneous H-systems with zero Dirichlet boundary conditions.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 52,
Issue. 1,
p.
239.
Liu, Yue
and
Wang, Zhengping
2007.
Biharmonic equations with asymptotically linear nonlinearities.
Acta Mathematica Scientia,
Vol. 27,
Issue. 3,
p.
549.
Wang, Weihua
and
Zhao, Peihao
2008.
Nonuniformly nonlinear elliptic equations of p-biharmonic type.
Journal of Mathematical Analysis and Applications,
Vol. 348,
Issue. 2,
p.
730.
Deng, Yin-bin
and
Wang, Li
2008.
Solutions for a class of singular nonlinear boundary value problem involving critical exponent.
Acta Mathematicae Applicatae Sinica, English Series,
Vol. 24,
Issue. 3,
p.
453.
Wang, Youjun
and
Shen, Yaotan
2009.
Multiple and sign-changing solutions for a class of semilinear biharmonic equation.
Journal of Differential Equations,
Vol. 246,
Issue. 8,
p.
3109.
Pei, Ruichang
2012.
Multiple solutions for a fourth-order nonlinear elliptic problem.
Boundary Value Problems,
Vol. 2012,
Issue. 1,
Xie, Huazhao
and
Wang, Jianping
2013.
Infinitely many solutions for p-harmonic equation with singular term.
Journal of Inequalities and Applications,
Vol. 2013,
Issue. 1,
FAN, Haining
and
LIU, Xiaochun
2014.
Multiple positive solutions for a class of quasi-linear elliptic equations involving critical sobolev exponent.
Acta Mathematica Scientia,
Vol. 34,
Issue. 4,
p.
1111.
Faria, Luiz F. O.
2015.
Existence and uniqueness of positive solutions for singular biharmonic elliptic systems.
p.
400.
Rani, Anu
and
Goyal, Sarika
2020.
Polyharmonic systems involving critical nonlinearities with sign-changing weight functions.
Electronic Journal of Differential Equations,
Vol. 2020,
Issue. 01-132,
p.
118.
Hoppe, Ronald H.W.
2022.
A C0 Interior Penalty Discontinuous Galerkin Method and an equilibrated a posteriori error estimator for a nonlinear fourth order elliptic boundary value problem of p-biharmonic type.
ESAIM: Mathematical Modelling and Numerical Analysis,
Vol. 56,
Issue. 6,
p.
2051.
Li, Qi
Han, Yuzhu
and
Wang, Tianlong
2023.
Existence and nonexistence of solutions to a critical biharmonic equation with logarithmic perturbation.
Journal of Differential Equations,
Vol. 365,
Issue. ,
p.
1.
Rani, Anu
and
Goyal, Sarika
2023.
Biharmonic system with Hartree-type critical nonlinearity.
Electronic Journal of Qualitative Theory of Differential
Equations,
p.
1.
Messirdi, Sofiane
Bennour, Abdelaziz
and
Matallah, Atika
2023.
On nonhomogeneous biharmonic problem involving Rellich-type potentials and a critical Sobolev exponent.
Indian Journal of Pure and Applied Mathematics,
Yu, Yang
and
Zhao, Yulin
2023.
Caffarelli–Kohn–Nirenberg inequality for biharmonic equations with inhomogeneous term and Rellich potential.
Electronic Journal of Qualitative Theory of Differential Equations,
p.
1.
Chen, Jianqing
and
Chen, Zhewen
2023.
Multiple Normalized Solutions for Biharmonic Choquard Equation with Hardy–Littlewood–Sobolev Upper Critical and Combined Nonlinearities.
The Journal of Geometric Analysis,
Vol. 33,
Issue. 12,
Hou, Songbo
2023.
Multiple Solutions of a Nonlinear Biharmonic Equation on Graphs.
Communications in Mathematics and Statistics,
Vol. 11,
Issue. 4,
p.
767.
Lei, Chunyu
Lei, Jun
and
Suo, Hongmin
2025.
Multiple solutions for a Bibarmonic elliptic equation with critical Choquard type nonlinearity.
Quaestiones Mathematicae,
Vol. 48,
Issue. 1,
p.
121.
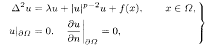
 ; Δ2 = ΔΔ denotes iterated N-dimensional Laplacian; f(x) is a given function. Some results on the existence and non-existence of multiple solutions for the above problem have been obtained by Ekeland's variational principle and the mountain-pass lemma under some assumptions on f(x) and N.
; Δ2 = ΔΔ denotes iterated N-dimensional Laplacian; f(x) is a given function. Some results on the existence and non-existence of multiple solutions for the above problem have been obtained by Ekeland's variational principle and the mountain-pass lemma under some assumptions on f(x) and N.