Let Ω ⊂ R2 denote a bounded Lipschitz domain and consider some portion Γ0 of ∂Ω representing the austenite–twinned-martensite interface which is not assumed to be a straight segment. We prove that 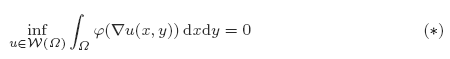for an elastic energy density ϖ: R2 → [0 ∞) such that ϖ(0, ±1) = 0. Here, W(Ω) consists of all functions u from the Sobolev class W1, ∞(Ω) such that |uy| = 1 almost everywhere on Ω together with u = 0 on Γ0. We will first show that, for Γ0 having a vertical tangent, one cannot always expect a finite surface energy, i.e. in the above problem, the condition in general cannot be included. This generalizes a result of [12] where Γ0is a vertical straight line. Property (*) is established by constructing some minimizing sequences vanishing on the whole boundary ∂Ω, that is, one can even take Γ0 = ∂Ω. We also show that the existence or non-existence of minimizers depends on the shape of the austenite–twinned-martensite interface Γ0.