No CrossRef data available.
Published online by Cambridge University Press: 24 January 2020
We are concerned with a nonnegative solution to the scalar field equation
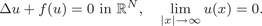 $$\Delta u + f(u) = 0{\rm in }{\open R}^N,\quad \mathop {\lim }\limits_{|x|\to \infty } u(x) = 0.$$
$$\Delta u + f(u) = 0{\rm in }{\open R}^N,\quad \mathop {\lim }\limits_{|x|\to \infty } u(x) = 0.$$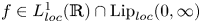 $f \in L^1_{loc}(\mathbb {R}) \cap \mathrm {Lip}_{loc}(0,\infty )$ with
$f \in L^1_{loc}(\mathbb {R}) \cap \mathrm {Lip}_{loc}(0,\infty )$ with  $\int _0^u f(s)\,{\rm d}s < 0$ for small
$\int _0^u f(s)\,{\rm d}s < 0$ for small 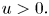 $u>0.$ Since they use a shooting argument for their proof, they require the property that
$u>0.$ Since they use a shooting argument for their proof, they require the property that 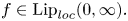 $f \in \mathrm {Lip}_{loc}(0,\infty ).$ In this paper, using a purely variational method, we extend the previous existence results for
$f \in \mathrm {Lip}_{loc}(0,\infty ).$ In this paper, using a purely variational method, we extend the previous existence results for 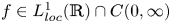 $f \in L^1_{loc}(\mathbb {R}) \cap C(0,\infty )$. We show that a solution obtained through minimization has the least energy among all radially symmetric weak solutions. Moreover, we describe a general condition under which a least energy solution has compact support.
$f \in L^1_{loc}(\mathbb {R}) \cap C(0,\infty )$. We show that a solution obtained through minimization has the least energy among all radially symmetric weak solutions. Moreover, we describe a general condition under which a least energy solution has compact support.