Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-12-01T02:11:30.275Z Has data issue: false hasContentIssue false
  • English
  • Français

I.—On Hamilton's Principal Function in Quantum Mechanics

Published online by Cambridge University Press:  14 February 2012

E. T. Whittaker
Get access Rights & Permissions [Opens in a new window]

Extract

The solution of a problem in classical dynamics can be described, as Hamilton showed in 1834, by its Principal Function. Considering for simplicity a conservative problem with one degree of freedom, let the co-ordinate at the instant t be q, and let the Lagrangean function be L. Let Q be the value of the co-ordinate at a previous instant T. Let the quantity 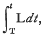, after the integration has been performed, be expressed in terms of (q, Q, t — T), and let the function thus obtained, which is Hamilton's Principal Function, be denoted by W.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 61 , Issue 1 , 1941 , pp. 1 - 19
Copyright
Copyright © Royal Society of Edinburgh 1941

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

page 1 note * More degrees of freedom complicate the mathematics without affecting the principles and methods involved.

page 3 note * Journ. für Math., vol. Ixvi, 1866, p. 161Google Scholar; cf. , Watson, Journ. Lond. Math. Soc, vol. viii, 1933, p. 194Google Scholar.

† For the benefit of those readers who are accustomed to use the notation for Hermite polynomials

‡ Myller-Lebedeff, W., Math. Ann., vol. lxiv, 1907, p. 388CrossRefGoogle Scholar; it has been rediscovered many times. Cf. , Hille, Proc. Nat. Acad. Sci. Washington, vol. xii, 1926, pp. 261, 265, 348Google Scholar; , Hardy, fourn. Lond. Math. Soc, vol. vii, 1932, pp. 138, 192Google Scholar.

page 4 note * We write the first term pgp rather than gp2 or p2g because pgp is Hermitean while gp2 and p2g are not Hermitean.

page 6 note * , Physik. Zeits. Sowjetunion, vol. iii, 1933, p. 64.Google Scholar

page 19 note * Watson, G. N., Bessel Functions, p. 395.Google Scholar