Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-05T14:34:19.716Z Has data issue: false hasContentIssue false
  • English
  • Français

Hankel operators with PC symbols and the space H∞ + PC

Published online by Cambridge University Press:  14 November 2011

F. F. Bonsall  and
T. A. Gillespie
F. F. Bonsall
Affiliation:
Department of Mathematics, University of Edinburgh
T. A. Gillespie
Affiliation:
Department of Mathematics, University of Edinburgh
Get access Rights & Permissions [Opens in a new window]

Synopsis

We obtain an explicit formula for the essential norm of a Hankel operator with its symbol in the space PC, which is the closure in L∞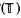 of the space of piecewise continuous functions on the unit circle 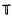. It follows from this formula that functions in PC can be approximated as closely by functions in C, the continuous functions on the circle, as by functions in the much larger space H∞ + C. This is an example of the way in which properties of the Hardy spaces can be derived from properties of Hankel operators.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 89 , Issue 1-2 , 1981 , pp. 17 - 24
Copyright
Copyright © Royal Society of Edinburgh 1981

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Adamjan, V. M., Arov, D. Z. and Krein, M. G.. Analytic properties of Schmidt pairs of a Hankel operator and the generalized Schur-Takagi problem. Mat. Sb. 86 (128), (1971), 34–75 (translated in Math. USSR-Sb. 15 (1971), 31–73).Google Scholar
2Carathéodory, C.. Theory of functions of a complex variable, Vol. II (New York: Chelsea, 1954).Google Scholar
3Douglas, R. G.. Banach algebra techniques in operator theory (New York: Academic Press, 1972).Google Scholar
4Gohberg, I. C. and Krupnik, N. Ja.. The algebra generated by Toeplitz matrices. Funkcional. Anal, i. Priložen. 3 No. 2 (1969), 45–56; Functional Anal. Appl. 3 (1969), 119–127.Google Scholar
5Hartman, P.. On completely continuous Hankel matrices. Proc. Amer. Math. Soc. 9 (1958), 862–866.Google Scholar
6Magnus, W.. On the spectrum of Hilbert's matrix. Amer. J. Math. 72 (1950), 699–704.Google Scholar
7Power, S. C.. The essential spectrum of a Hankel operator with piecewise continuous symbol. Michigan Math. J. 25 (1978), 117–121.CrossRefGoogle Scholar
8Power, S. C.. Hankel operators on Hilbert space. Bull. London Math. Soc. 12 (1980), 422–442.Google Scholar
9Sarason, D.. Approximation of piecewise continuous functions by quotients of bounded analytic functions. Canadian J. Math. 24 (1972), 642–657.Google Scholar