Article contents
Geršgorin theory and the definiteness of determinantal operators
Published online by Cambridge University Press: 14 November 2011
Synopsis
Let Aij, l≦j≦k, be bounded Hermitean operators on Hilbert spaces Hi, 1≦i≦k, and let 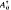 be the induced operators on
be the induced operators on 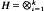 . An important operator for multiparameter theory is δ: H →H denned by δ = det
. An important operator for multiparameter theory is δ: H →H denned by δ = det 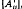 the determinant being expanded formally. Various definiteness properties of δ are critical for multiparameter spectral theory.
the determinant being expanded formally. Various definiteness properties of δ are critical for multiparameter spectral theory.
We use the operators Aij to construct a numerical matrix δ(δ) upon which we use Geršgorin theory to investigate the non-singularity and definiteness of δ. Diagonal dominance properties of the array [Aij] are also discussed.
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 106 , Issue 1-2 , 1987 , pp. 1 - 10
- Copyright
- Copyright © Royal Society of Edinburgh 1987
References
- 1
- Cited by


