Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-19T03:57:46.928Z Has data issue: false hasContentIssue false
  • English
  • Français

Existence conditions for higher order eigensets of multiparameter operators

Published online by Cambridge University Press:  14 November 2011

Paul Binding ,
Patrick J. Browne  and
Lawrence Turyn
Paul Binding
Affiliation:
Department of Mathematics and Statistics, University of Calgary, Calgary, Alberta, CanadaT2N 1N4
Patrick J. Browne
Affiliation:
Department of Mathematics and Statistics, University of Calgary, Calgary, Alberta, CanadaT2N 1N4
Lawrence Turyn
Affiliation:
Department of Mathematics and Statistics, Wright State University, Dayton, Ohio 45435, U.S.A.
Get access Rights & Permissions [Opens in a new window]

Synopsis

We consider classes of self-adjoint operators 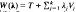for which the nonpositive part of the spectrum consists of eigenvalues ρ0(λ)≦ ρ1(») ≦ … repeated according to multiplicity. The sets of λ where ρi(λ) is negative and zero are labelled Ni and Zi respectively, and Pi = ℝk\(Ni ∪ Zi). We study conditions on the Vj sufficient to ensure nonemptiness of at least one of Ni, Zi and Pi for all T or for all positive definite T, as well as conditions which are necessary in the sense that failure permits emptiness for at least one T.

As an example of our results, we show in the Sturm–Liouville case

with L∞ coefficients and separated end conditions, that nonemptiness of Zi for all T (i.e. for all p > 0, all q and all boundary data) is equivalent to the i-independent condition that the ftj do not vanish simultaneously on a set of positive measure.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 103 , Issue 1-2 , 1986 , pp. 137 - 146
Copyright
Copyright © Royal Society of Edinburgh 1986

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Binding, P. A.. Dual variational approaches to multiparameter eigenvalue problems. J. Math. Anal. Appl. 92 (1983), 96–113.Google Scholar
2Binding, P. A. and Browne, P. J.. Spectral properties of two parameter eigenvalue problems. Proc. Roy. Soc. Edinburgh Sect. A 89 (1981), 157–173.CrossRefGoogle Scholar
3Binding, P. A., Browne, P. J. and Turyn, L.. Existence conditions for two-parameter eigenvalue problems. Proc. Roy. Soc. Edinburgh Sect. A 91 (1981), 15–30.CrossRefGoogle Scholar
4Binding, P. A., Browne, P. J. and Turyn, L.. Existence conditions for eigenvalue problems generated by compact multiparameter operators. Proc. Roy. Soc. Edinburgh Sect. A 96 (1984), 261–274.CrossRefGoogle Scholar
5Binding, P. A., Browne, P. J. and Turyn, L.. Spectral properties of compact multiparameter operators. Proc. Roy. Soc. Edinburgh Sect. A 98 (1984), 291–303.Google Scholar
6Richardson, R. G.. Theorems of oscillation for two linear differential equations of the second order with two parameters. Trans. Amer. Math. Soc. 13 (1912), 22–34.CrossRefGoogle Scholar
7Richardson, R. G.. Über die notwendig und hinreichenden Bedingungen für Bestehen eines Kleinschen Oszillationstheorems. Math. Ann. 73 (1912/1913), 289–304.Google Scholar
8Rockafellar, R. T.. Convex Analysis (Princeton: Princeton University Press, 1972).Google Scholar
9Schafke, R. and Volkmer, H.. A note on the paper ‘Existence conditions for eigenvalue problems generated by compact multiparameter operators’ by Binding, P., Browne, P. J. and Turyn, L.. Proc. Roy. Soc. Edinburgh Sect. A 101 (1985), 147–148.CrossRefGoogle Scholar
10Turyn, L.. Sturm–Liouville problems with several parameters. J. Differential Equations 38 (1980), 239–259.Google Scholar
11Zaanen, A. C.. Integration (Amsterdam: North Holland, 1967).Google Scholar