Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Rankin, R. A.
1986.
Fourier coefficients of cusp forms.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 100,
Issue. 1,
p.
5.
Rankin, R. A.
2000.
Number Theory.
p.
371.
Chan, Heng Huat
2007.
Triple product identity, Quintuple product identity and Ramanujan’s differential equations for the classical Eisenstein series.
Proceedings of the American Mathematical Society,
Vol. 135,
Issue. 7,
p.
1987.
Fukuhara, Shinji
2010.
The elliptic Apostol–Dedekind sums generate odd Dedekind symbols with Laurent polynomial reciprocity laws.
Mathematische Annalen,
Vol. 346,
Issue. 4,
p.
769.
Maier, Robert S.
2011.
Nonlinear differential equations satisfied by certain classical modular forms.
Manuscripta Mathematica,
Vol. 134,
Issue. 1-2,
p.
1.
Kim, Daeyeoul
Kim, Aeran
and
Park, Hwasin
2013.
CONGRUENCES OF THE WEIERSTRASS <TEX>${\wp}(x)$</TEX> AND <TEX>${\wp}^{{\prime}{\prime}}(x)$</TEX>(<TEX>$x=\frac{1}{2}$</TEX>, <TEX>$\frac{\tau}{2}$</TEX>, <TEX>$\frac{\tau+1}{2}$</TEX>)-FUNCTIONS ON DIVISORS.
Bulletin of the Korean Mathematical Society,
Vol. 50,
Issue. 1,
p.
241.


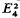 , relating these series. These are usually proved by arguments making use of the dimensions of vector spaces of modular forms, and not directly. The paper shows how such identities can be proved by elementary methods by studying chains of solutions of Diophantine equations of the form xξ+
, relating these series. These are usually proved by arguments making use of the dimensions of vector spaces of modular forms, and not directly. The paper shows how such identities can be proved by elementary methods by studying chains of solutions of Diophantine equations of the form xξ+