Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-19T05:54:26.871Z Has data issue: false hasContentIssue false
  • English
  • Français

Complex and real singularities of eigenfunction expansions

Published online by Cambridge University Press:  14 November 2011

Ahmed I. Zayed
Ahmed I. Zayed
Affiliation:
Mathematics Department, California Polytechnic State University, San Luis Obispo, CA 93407, U.S.A.
Get access Rights & Permissions [Opens in a new window]

Synopsis

Let θ(t, λ) and (t,λ) be the solutions to the differential equation y“+(λ − q(t))y =0, − ∞ <t < ∞ such that θ(0, λ)) = l, θ'(0, λ)) = 0, ø(0, λ) = 0 and ø'(0, λ) = −1. It is known that for a function f(t)∈ L2(−∞,∞), we have

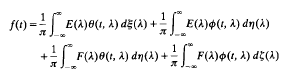
where 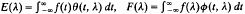for some measures dξ, dη; and dζ independent of f.

In the first part of this paper we devise a technique to locate the singular points of f(t) (t is complex) under the assumptions that E(λ), F(λ) are of order O(e-cvλ), as λ →∞and q(t) is a sufficiently nice function. In this case all the singularities of fare off the real axis. In the second part of the paper we relax the restriction on E(λ) and f(λ) so that they are of order O(λk) as λ →∞ for some constant k, and we show that in this case f(t) is a generalised function whose analytic representation is holomorphic in the upper and lower half planes. We then devise a technique to continue analytically across the real axis and locate its singularities thereon.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 103 , Issue 3-4 , 1986 , pp. 179 - 199
Copyright
Copyright © Royal Society of Edinburgh 1986

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Bremermann, H.. Distributions, Complex variables and Fourier transforms (New York: Addison Wesley, 1965).Google Scholar
2Gilbert, R.. Bergman's integral operator method in generalized axially symmetric potential theory. J. Math. Phys. 5 (1964), 983–997.CrossRefGoogle Scholar
3Gilbert, R.. Function Theoretic Methods in Partial Differential Equations (New York:Academic Press, 1969).Google Scholar
4Gilbert, R. and Howard, H.. A generalization of a theorem of Nehari. Bull. Amer. Math. Soc. 72 (1966), 37–43.CrossRefGoogle Scholar
5Gilbert, R. and Howard, H.. The scope of the function theoretic approach for equations permitting a separation of variables. J. Math. Anal. Appl. 34 (1971), 671–684.CrossRefGoogle Scholar
6Levitan, B. and Sargsjan, I.. Introduction to spectral theory. Mathematical Monographs 39 (Providence, R.I.: Amer. Math. Soc, 1975).Google Scholar
7Levitan, B.. An inverse problem for the Sturm-Liouville operator in the case of finite-zone and infinite-zone potentials. Trans. Moscow Math. Soc. 45 (1984), 1–34 (Providence, R.I.: Amer. Math. Soc).Google Scholar
8Nehari, Z.. On the singularities of Legendre expansions. J. Rational Mech. Anal. 5 (1956), 987–992.Google Scholar
9Newton, R.. Scattering theory of waves and particles. (New York: McGraw-Hill. 1966).Google Scholar
10Omnes, R. and Froissart, M.. Mendelstam theory and Regge poles (New York:Benjamin, 1963).Google Scholar
11. Strakhov, V.. The analytic continuation of two dimensional potential fields with applications to the solution of the inverse problems of magnetic and gravitational exploration, I, II, III, IZV. Geophys. ser. (English transl.) (1962), 209–214, 227–232, 324–331.Google Scholar
12Szegö, G.. On the singularities of zonal harmonic expansions. J. Rational Mech. Anal. 3 (1954), 561–564.Google Scholar
13Titchmarsh, E.. Eigenfunction expansions associated with second-order differential equations (Oxford: Oxford University Press, 1962).CrossRefGoogle Scholar
14Walter, G.. On real singularities of Legendre expansions. Proc. Amer. Math. Soc. 19 (1968), 1407–1412.CrossRefGoogle Scholar
15Zayed, A.. On the singularities of Gegenbauer (ultraspherical) expansions. Trans. Amer. Math. Soc. 262 (1980), 487–503.CrossRefGoogle Scholar
16Zayed, A. and Walter, G.. Series of orthogonal polynomials as hyperfunctions. SIAM J. Math. Anal. 13 (1982), 664–675.CrossRefGoogle Scholar
17Zayed, A. and Walter, G.. On the singularities of singular Sturm-Liouville expansions andan associated class of elliptic P.D.E.'s. SIAM J. Math. Anal. 16 (1985), 725–740.CrossRefGoogle Scholar
18Zemanian, A.. Generalised Integral transformations (New York: Wiley Interscience, 1968).Google Scholar