Hostname: page-component-745bb68f8f-5r2nc Total loading time: 0 Render date: 2025-02-05T14:16:05.621Z Has data issue: false hasContentIssue false
  • English
  • Français

31.—Spectral Manifolds for Constant Coefficient Elliptic Operators in Lp(Rn)*

Published online by Cambridge University Press:  14 February 2012

M. Thompson
M. Thompson
Affiliation:
Mathematics Division, University of Sussex.
Get access Rights & Permissions [Opens in a new window]

Synopsis

The principal results of this paper concern the spectral properties of the maximal realisation Pp in Lp(Rn) of a formally self adjoint constant coefficient strongly elliptic partial differential operator P(D), assumed to be homogeneous of order 2m, for 1 ≦ p ≦ ∞ and n ≧ 2. If we assume that 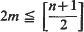, for 2n/n + l ≦ p ≦ 2n/n−1, together with certain assumptions on the associated real zero surfaces P(ξ) = λ, λ > 0, then σ(Pp) = σc(Pp) = [0, ∞). We obtain an estimate on the norm of the resolvent of Pp for points near the real axis, which allows us to establish the existence of a generalised resolution of the identity in the sense of Kocan.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 72 , Issue 4 , 1975 , pp. 359 - 369
Copyright
Copyright © Royal Society of Edinburgh 1975

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

References to Literature

[1] Balslev, E., 1965. The essential spectrum of elliptic differential operators in L p(R n). Trans. Am. Math. Soc., 116, 193–217.Google Scholar
[2] Bartle, R. G., 1970. Spectral decomposition of operators in Banach spaces. Proc. Lond. Math. Soc., 20, 438–50.Google Scholar
[3] Dunford, N. and Schwartz, J. T., 1958. Linear Operators, I. New York: Interscience.Google Scholar
[4] Fefferman, C., 1971. The multiplier for the ball. Ann. Math., 94, 330–336.CrossRefGoogle Scholar
[5] John, F., 1955. Plane waves and spherical means applied to partial differential equations. New York: Interscience.Google Scholar
[6] Kato, T., 1960. Note on fractional powers of linear operators. Proc. Jap. Acad., 36, 94–96.Google Scholar
[7] Kocan, D., 1966. Spectral manifolds for a class of operators. Illinois J. Math., 10, 605–622.Google Scholar
[8] Komatsu, H., 1964. Semigroups of operators in locally convex spaces. J. Math. Soc. Japan., 16, 230–262.CrossRefGoogle Scholar
[9] Komatsu, H., 1969. Fractional powers of operators. Pacif. J. Math., 19, 285–346.Google Scholar
[10] Murray, F. J., 1945. Quasi-complements and closed projections in reflexive Banach spaces. Trans. Am. Math. Soc., 58, 77–95.Google Scholar
[11] Talenti, G., 1971. Spectrum of the Laplace operator acting in Lp(Rn). Symp. Math., 7. Bologna: 1st. Naz. Alta Mat.Google Scholar
[12] Thompson, M., 1972. Eigenfunction expansions and scattering theory for perturbed elliptic partial differential equations. Communs Pure Appl. Math., 25, 499–532.CrossRefGoogle Scholar
[13] Vainberg, B. R., 1966. Principles of radiation, limit absorption and limit amplitude in the general theory of partial differential equations. Russ. Math. Survs, 21, 115–193.CrossRefGoogle Scholar