Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-26T01:27:00.093Z Has data issue: false hasContentIssue false
  • English
  • Français

18.—Singular Linear Differential Operators with many Parameters*

Published online by Cambridge University Press:  14 February 2012

B. D. Sleeman
B. D. Sleeman
Affiliation:
Department of Mathematics, University of Dundee
Get access Rights & Permissions [Opens in a new window]

Synopsis

It is the purpose of this paper to make a study of the solutions of the following k-formally self-adjoint differential equations

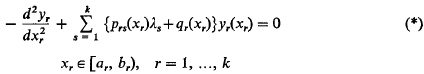
where ar, br), r = 1, 2, …, k, denote k semi-open intervals in which ar is finite and br is arbitrary and the λs, s = 1, 2, …, k, are spectral parameters.

The main theme of the paper is that of extending the Hermann Weyl limit-point, limit-circle theory to the multi-parameter case. That is we consider under which circumstances there exist, for each r, one or two solutions yr(xr) of (*) which are square integrable in a suitably defined Hilbert space Hr. This is then generalised to consider the problem of investigating the possibility of the product

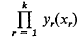
of solutions of (*) being square integrable in H, the tensor product of the separate spaces Hr. The analyticky of the corresponding generalised Hermann Weyl coefficients mr(λ1, λ2,…, λk), r = 1,…, k, is also investigated. Some examples illustrating the theory are given and an alternative formulation of the problem is suggested.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 71 , Issue 3 , 1973 , pp. 199 - 232
Copyright
Copyright © Royal Society of Edinburgh 1973

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

References to Literature

Akhiezer, N. I. and Glazman, I. H., 1963. Theory of Linear Operators in Hilbert Space, Vols. I and II. New York: Ungar.Google Scholar
Arscott, F. M., 1964 a. Periodic Differential Equations. London: Pergamon.Google Scholar
Arscott, F. M., 1964 b. Two-parameter eigenvalue problems in differential equations. Proc. Land. Math. soc., 14, 459–470.Google Scholar
Atkinson, F. V., 1968. Multi-parameter spectral theory. Bull. Am. Math. soc., 74, 1–28.CrossRefGoogle Scholar
Browne, P. J., 1972 a. A multi-parameter eigenvalue problem. J. Math. Analysis Applic, 38, 553–568.Google Scholar
Browne, P. J., 1972 b. A singular multi-parameter eigenvalue problem in second order ordinary differential equations. J. Diff. Equations, 12, 81–94.Google Scholar
Coddington, E. A. and Levinson, N., 1955. Theory of Ordinary Differential Equations. New York: McGraw-Hill.Google Scholar
Cordes, H. O., 1954–1955. Uber die Spektralzerlegung von hypermaximalen operatoren die durch separaten der variablen zerfallen, I. Math. Annin, 128, 257–289, and II, Math. Annin, 129, 373–411.CrossRefGoogle Scholar
Dugundn, J., 1966. Topology. Boston: Allyn and Bacon.Google Scholar
Dunford, N. and Schwarz, J. T., 1964. Linear Operators. New York: Interscience.Google Scholar
Erdélyi, A.et al., 1953. Higher Transcendental Functions, Vol. III. New York: McGraw-Hill.Google Scholar
Evans, W. D., 1971. On the limit-point, limit-circle classification of a second order differential equation with a complex coefficient. J. Lond. Math. soc., 4, 245–256.CrossRefGoogle Scholar
Faierman, M., 1969. The completeness and expansion theorems associated with the multi-parameter eigenvalue problem in ordinary differential equations. J. Diff. Equations, 5, 197–213.CrossRefGoogle Scholar
Gunning, R. and Rossi, H., 1965. Analytic Functions of Several Complex Variables. London: Prentice-Hall.Google Scholar
Hellwig, G., 1964. Differential Operators of Mathematical Physics. London: Addison Wesley.CrossRefGoogle Scholar
Hill, G. W., 1886. On the part of the motion of the lunar perigee. Acta Math., Stockh., 8, 1–36.CrossRefGoogle Scholar
Ince, E. L., 1926. Ordinary Differential Equations. London: Longmans Green.Google Scholar
Magnus, W. and Winkler, S., 1966. Hil's Equation. New York: Interscience.Google Scholar
Mekner, J. and Schafke, F. W., 1954. Mathieusche Funktionen und Spharoid-funktionen. Berlin: Springer.Google Scholar
Miller, K., 1964. Linear Differential Equations. London: Routledge and Kegan Paul.Google Scholar
Murray, F. J. and Von Neumann, J., 1936. On rings of operators. Ann. Math., 37, 116–229.CrossRefGoogle Scholar
Naimark, M. A., 1968. Linear Differential Operators. London: Harrap.Google Scholar
Richardson, R. G. D., 1912. Theorems of oscillation for two linear differential equations of the second order with two parameters. Trans. Am. Math. soc., 13, 22–34.Google Scholar
Sim, A. R., 1957. Secondary conditions for linear differential operators of the second order. J. Math. Mech., 6, 247–285.Google Scholar
Sleeman, B. D., 1971. Multi-parameter eigenvalue problems in ordinary differential equations. Bul. Inst. Politeh. Iasi, (1), 17 (21), 51–60.Google Scholar
Sleeman, B. D., 1972. Completeness and expansion theorems for a two-parameter eigenvalue problem in ordinary differential equations using variational principles. J. Land. Math. Soc. (in press).Google Scholar
Sleeman, B. D. and Kallstrom, A., 1972. Completeness and expansion theorems for the multi-parameter eigenvalue problem in ordinary differential equations (in press).Google Scholar
Titchmarsh, E. C., 1962. Eigenfunction Expansions associated with Second Order Differential Equations, 2nd edn.O.U.P.CrossRefGoogle Scholar
Whtttaker, E. T. and Watson, G. N., 1962. Modern Analysis. C.U.P.Google Scholar