We study the dynamics of a two-planet system, which evolves being in a 1/1 mean motion resonance (co-orbital motion) with non-zero mutual inclination. In particular, we examine the existence of bifurcations of periodic orbits from the planar to the spatial case. We find that such bifurcations exist only for planetary mass ratios 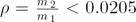$\rho=\frac{m_2}{m_1}<0.0205$. For ρ in the interval 0<ρ<0.0205, we compute the generated families of spatial periodic orbits and their linear stability. These spatial families form bridges, which start and end at the same planar family. Along them the mutual planetary inclination varies. We construct maps of dynamical stability and show the existence of regions of regular orbits in phase space.