Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-23T13:38:30.327Z Has data issue: false hasContentIssue false
  • English
  • Français

Weighted Estimates for Solutions of the General Sturm-Liouville Equation and the Everitt-Giertz Problem. I

Part of: Qualitative theory General theory in ordinary differential equations

Published online by Cambridge University Press:  21 July 2014

N. A. Chernyavskaya  and
L. A. Shuster
N. A. Chernyavskaya
Affiliation:
Department of Mathematics and Computer Science, Ben-Gurion University of the Negev, PO Box 653, Beer-Sheva 84105, Israel
L. A. Shuster
Affiliation:
Department of Mathematics, Bar-Ilan University, 52900 Ramat Gan, Israel, ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

Consider the equation

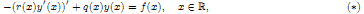
where ƒ ∈ Lp(ℝ), p ∈ (1, ∞) and

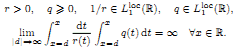
By a solution of (*), we mean any function y absolutely continuous together with (ry′) and satisfying (*) almost everywhere on ℝ. In addition, we assume that (*) is correctly solvable in the space Lp(ℝ), i.e.

(1) for any function 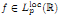, there exists a unique solution y ∈ Lp(ℝ) of (*);

(2) there exists an absolute constant c1(p) > 0 such that the solution y ∈ Lp(ℝ) of (*) satisfies the inequality

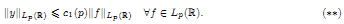
We study the following problem on the strengthening estimate (**). Let a non-negative function 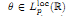be given. We have to find minimal additional restrictions for θ under which the following inequality holds:

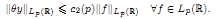
Here, y is a solution of (*) from the class Lp(ℝ), and c2 (p) is an absolute positive constant.

Keywords

linear differential equationssecond-order equationEveritt—Giertz problem

MSC classification

Primary: 34C10: Oscillation theory, zeros, disconjugacy and comparison theory
Secondary: 34A99: None of the above, but in this section
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 58 , Issue 1 , February 2015 , pp. 125 - 147
Copyright
Copyright © Edinburgh Mathematical Society 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1.Amanova, T. T., On the separability of a differential operator, Izv. Akad. Nauk Kazakh. SSR Ser. Fiz.-Mat. 3 (1981), 48–51.Google Scholar
2.Atkinson, F. V., On some results of Everitt and Giertz, Proc. R. Soc. Edinb. A 71 (1973), 151–158.Google Scholar
3.Baĭdel′dinov, B. L., On the problem of the solvability of the singular Sturm-Liouville equation in weighted Lp-class, Izv. Akad. Nauk Kazakh. SSR Ser. Fiz.-Mat. 5 (1991), 15–20.Google Scholar
4.Birgebaev, A., Separability of a differential operator in Lp Izv. Akad. Nauk Kazakh. SSR Ser. Fiz.-Mat. 5 (1981), 1–5.Google Scholar
5.Birgebaev, A. and Otelbaev, M., Separability of a third-order nonlinear differential operator, Izv. Akad. Nauk Kazakh. SSR Ser. Fiz.-Mat. 3 (1984), 11–13.Google Scholar
6.Boimatov, K. H., Separability theorems for the Sturm-Liouville operator, Mat. Zametki 14 (1973), 349–359.Google Scholar
7.Boimatov, K. H., Theorems on the separation property, Dokl. Akad. Nauk SSSR 213 (1973), 1009–1011.Google Scholar
8.Boimatov, K. H., The domain of definition of a Sturm—Liouville operator, Diff. Uravn. 12(7) (1976), 1151–1160.Google Scholar
9.Boimatov, K. H., Separability theorems, weighted spaces and their application to boundary value problems, Dokl. Akad. Nauk SSSR 247(3) (1979), 532–536.Google Scholar
10.Boimatov, K. K., Separation theorems, weighted spaces and their applications, in Studies in the theory of differentiable functions of several variables and its applications, X, Proceedings of the Steklov Institute of Mathematics, Volume 156, pp. 37–36 (American Mathematical Society, Providence, RI, 1984).Google Scholar
11.Boimatov, K. K., Coercive estimates and separability for second-order elliptic differential equations, Dokl. Akad. Nauk SSSR 301(5) (1988), 1033–1036.Google Scholar
12.Boimatov, K. K., Coercive estimates and separability for second-order nonlinear elliptic differential operators, Mat. Zametki 46(6) (1989), 110–112.Google Scholar
13.Boimatov, K. K. and Lizorkin, P. I., Estimates for the growth of solutions of differential equations, Diff. Uravn. 25(4) (1989), 578–588.Google Scholar
14.Boimatov, K. K. and Sharifov, A., Coercive estimates and separability for differential operators of arbitrary order, Russ. Math. Surv. 44(3) (1989), 147–148.CrossRefGoogle Scholar
15.Brown, R. C., Separation and disconjugacy, J. Ineq. Pure Appl. Math. 4(3) (2003), 56.Google Scholar
16.Brown, R. C. and Cook, J., Continuous invertibility of minimal Sturm—Liouville operators in Lebesgue spaces, Proc. R. Soc. Edinb. A 136 (2006), 53–70.Google Scholar
17.Brown, R. C. and Hinton, D. B., Two separation criteria for second order ordinary or partial differential operators, Math. Bohem. 124(2) (1999), 273–292.CrossRefGoogle Scholar
18.Brown, R. C., Hinton, D. B. and Shaw, M. F., Some separation criteria and inequalities associated with linear second order differential operators, in Function spaces and applications, pp. 7–35 (Chemical Rubber Company, Boca Raton, FL, 2000).Google Scholar
19.Bulabaev, A. and Shuster, L., Summability with a weight of solutions of the Sturm-Liouville equation in Lp, in Functional analysis, differential equations and their applications, Volume 11-16 (Kazakh Gos. Univ., Alma-Ata, 1987).Google Scholar
20.Bulabaev, A., Otelbaev, M. and Shuster, L., Properties of Green's function of a Sturm-Liouville operator and their applications, Diff. Eqns 25(7) (1989), 773–779.Google Scholar
21.Chernyavskaya, N., Conditions for correct solvability of a simplest singular boundary value boundary problem, Math. Nachr. 243 (2002), 5–18.Google Scholar
22.Chernyavskaya, N. and Shuster, L., Estimates for the Green function of a general Sturm-Liouville operator and their applications, Proc. Am. Math. Soc. 127(5) (1999), 1413–1426.CrossRefGoogle Scholar
23.Chernyavskaya, N. and Shuster, L., Weight summability of solutions of the Sturm-Liouville equation, J. Diff. Eqns 151(2) (1999), 456–473.CrossRefGoogle Scholar
24.Chernyavskaya, N. and Shuster, L., Conditions for correct solvability of a simplest singular boundary value problem of general form, I, Z. Analysis Anwend. 7(1) (2000), 65–84.Google Scholar
25.Chernyavskaya, N. and Shuster, L., Regularity of the inverse problem for a Sturm-Liouville equation in Lp(R), Meth. Applic. Analysis 25 (2006), 205–235.Google Scholar
26.Chernyavskaya, N. and Shuster, L., A criterion for correct solvability in Lp(R) of a general Sturm-Liouville equation, J. Lond. Math. Soc. 80(2) (2009), 99–120.Google Scholar
27.Chernyavskaya, N. and Shuster, L., A criterion for compactness in Lp(R) of the resolvent of the maximal Sturm-Liouville operator of general form, preprint (arXiv:0912.0359, 2009).Google Scholar
28.Chernyavskaya, N. and Shuster, L., Weight estimates for solutions of linear singular differential equations of the first order and the Everitt-Giertz problem, Diff. Integ. Eqns 25(5) (2012), 467–504.Google Scholar
29.Chernyavskaya, N., El-Natanov, N. and Shuster, L., Weighted estimates for solutions of a Sturm-Louiville equation in the space L 1(R), in Proceedings of the fifth international conference on mathematical modeling and computer simulation of material technologies, MMT-2008: Ariel University Center of Samaria, Ariel, Israel, September 8-12, Volume 2, pp. 121–123 (Ariel University Center of Samaria, 2008).Google Scholar
30.Chernyavskaya, N., El-Natanov, N. and Shuster, L., Weighted estimates for solutions of a Sturm-Louiville equation in the space L 1(R), Proc. R. Soc. Edinb. A 141 (2011), 1175–1206.Google Scholar
31.Courant, R., Partial differential equations (Wiley, 1962).Google Scholar
32.Davies, E. B. and Harrell, E. M., Conformally flat Riemannian metrices, Schrödinger operators and semi-classical approximation, J. Diff. Eqns 66(2) (1987), 165–188.Google Scholar
33.Dzhumabaev, D. S. and Medetbekova, R. A., Separability of a second-order linear differential operator, Izv. Akad. Nauk Kazakh. SSR Ser. Fiz.-Mat. 5 (1983), 21–26.Google Scholar
34.Evans, W. D. and Zettl, A., Dirichlet and separation results for Schrödinger-type operators, Proc. R. Soc. Edinb. A 80 (1978), 151–162.Google Scholar
35.Everitt, W. N. and Giertz, M., Some properties of the domains of certain differential operators, Proc. Lond. Math. Soc. 23(3) (1971), 301–324.Google Scholar
36.Everitt, W. N. and Giertz, M., Some inequalities associated with certain differential operators, Math. Z. 126 (1972), 308–326.Google Scholar
37.Everitt, W. N. and Giertz, M., On some properties of the domains of the powers of formally self-adjoint differential expressions, Proc. Lond. Math. Soc. 24(3) (1972), 756–768.Google Scholar
38.Everitt, W. N. and Giertz, M., An example concerning the separation properties of differential operators, Proc. R. Soc. Edinb. A 71 (1972), 159–165.Google Scholar
39.Everitt, W. N. and Giertz, M., On limit point and separation criteria for linear differential expressions, in Proceedings of Equadiff III, 3rd Czechoslovak conference on differential equations and their applications, Brno, Czechoslovakia, August 28–September 1, 1972, pp. 31–41 (Czechoslovak Academy of Sciences, 1972).Google Scholar
40.Everitt, W. N. and Giertz, M., A Dirichlet type result for ordinary differential operators, Math. Ann. 203 (1973), 119–128.Google Scholar
41.Everitt, W. N. and Giertz, M., Inequalities and separation for certain ordinary differential operators, Proc. Lond. Math. Soc. 28(3) (1974), 352–372.Google Scholar
42.Everitt, W. N. and Giertz, M., On certain ordinary differential expressions and assoicated integral inequalities, in New developments in differential equations, North-Holland Mathematics Studies, Volume 21, pp. 161–174 (North-Holland, Amsterdam, 1976).Google Scholar
43.Everitt, W. N. and Giertz, M., Inequalities and separation for Schrodinger type operators in L 2(Rn), Proc. R. Soc. Edinb. A 79(3) (1978), 257–265.Google Scholar
44.Everitt, W. N., Giertz, M. and Weidmann, J., Some remarks on a separation and limit-point criterion of second order ordinary differential expressions, Math. Ann. 200 (1973), 335–346.Google Scholar
45.Grinshpun, E. Z. and Otelbaev, M., Smoothness of solutions of a nonlinear Sturm-Liouville equation in L 1(− ∞, ∞), Izv. Akad. Nauk Kazakh. SSR Ser. Fiz.-Mat. 5 (1984), 26–29.Google Scholar
46.Izmaylov, A. L., Smoothness of solutions of differential equations and separability differential equations, PhD thesis, Alma-Ata (1978).Google Scholar
47.Izmaylov, A. L. and Otelbaev, M., Summability with weight of the solution of a differential equation in an unbounded domain, Izv. Akad. Nauk Kazakh. SSR Ser. Fiz.-Mat. 1 (1977), 36–40.Google Scholar
48.Kufner, A. and Persson, L. E., Weighted inequalities of Hardy type (World Scientific, 2003).Google Scholar
49.Lukachev, M. and Shuster, L., On uniqueness of the solution of a linear differential equation without boundary conditions, Funct. Diff. Eqns 14 (2007), 337–346.Google Scholar
50.Mynbaev, K. and Otelbaev, M., Spectrum, coercivity, boundary value problem for differential equations and related problems of functional analysis, in Applied problems of mathematical physics and functional analysis, Volumes 167-168, pp. 81–88 (Nauka, Moscow, 1985).Google Scholar
51.Mynbaev, K. and Otelbaev, M., Weighted function spaces and the spectrum of differential operators (Nauka, Moscow, 1988).Google Scholar
52.Oinarov, R., Separability of the Schrödinger operator in the space of summable functions, Dokl. Akad. Nauk SSSR 285(5) (1985), 1062–1064.Google Scholar
53.Oinarov, R., Properties of Sturm—Liouville operator in Lp, Izv. Akad. Nauk Kazakh. SSR 1 (1990), 43–47.Google Scholar
54.Otelbaev, M., The summability with weight of the solution of a Sturm—Liouville equation, Mat. Zametki 6(6) (1974), 969–980.Google Scholar
55.Otelbaev, M., The separation of elliptic operators, Dokl. Akad. Nauk SSR Ser. Fiz.-Mat. 234(3) (1977), 540–543.Google Scholar
56.Otelbaev, M., The smoothness of the solution of differential equations, Izv. Akad. Nauk. Kazakh SSR 5 (1977), 45–48.Google Scholar
57.Otelbaev, M., A criterion for the resolvent of a Sturm-Liouville operator to be a kernel, Math. Notes 25 (1979), 296–297.Google Scholar
58.Otelbaev, M., Coercive estimates and separability theorems for elliptic equations in Lp(R), in Studies in the theory of differentiable functions of several variables and its applications, IX, Proceedings of the Steklov Institute of Mathematics, Volume 161, pp. 195–217 (American Mathematical Society, Providence, RI, 1984).Google Scholar
59.Ž. Raîmbekov, D., Smoothness of the solution in L 2 singular equation, Izv. Akad. Nauk Kazak. SSR Ser. Fiz.-Mat. 3 (1974), 78–83.Google Scholar
60.Sapenov, M. and Shuster, L. A., On the summability with weight of the solutions of binomial differential equations, Izv. Akad. Nauk Kazakh. SSR Ser. Fiz.-Mat. 1 (1987), 38–42.Google Scholar
61.Sapenov, M. and Shuster, L. A., Estimates of Green's function and a theorem on separability of a Sturm-Liouville operator in Lp (1985), manuscript available at VINITI, No. 8257-B85.Google Scholar
62.Togochuev, A. Z., Summability of the solution of a differential equation of odd order with weight, Izv. Akad. Nauk Kazakh. SSR Ser. Fiz.-Mat. 5 (1985), 55–58.Google Scholar
63.Zettl, A., Separation for differential operators and the Lp-spaces, Proc. Am. Math. Soc. 55(1) (1976), 44–46.Google Scholar