Published online by Cambridge University Press: 20 January 2009
Soit Θ un automorphisme analytique de 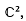 , Θ ayant un point fixe attractif zo (c'está-dire il existe un voisinage V de zo tel que pour tout élément z de V, on ait
, Θ ayant un point fixe attractif zo (c'está-dire il existe un voisinage V de zo tel que pour tout élément z de V, on ait 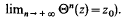 Alors d'aprés un résultat bien connu, il existe une fonction F entiére de
Alors d'aprés un résultat bien connu, il existe une fonction F entiére de 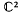 dans
dans 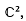 , injective, telle que
, injective, telle que 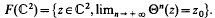 Ceci provient du fait qu'il existe une fonction F, définie au voisinage de z0, telle que F'(zo)=I, vérifiant Fequation fonctionnelle
Ceci provient du fait qu'il existe une fonction F, définie au voisinage de z0, telle que F'(zo)=I, vérifiant Fequation fonctionnelle 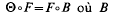 est un automorphisme analytique de
est un automorphisme analytique de 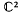 vérifiant
vérifiant  pour tout z élément de
pour tout z élément de 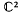 .
.