Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-24T13:11:07.262Z Has data issue: false hasContentIssue false
  • English
  • Français

Summability of alternating gap series

Published online by Cambridge University Press:  20 January 2009

J. P. Keating  and
J. B. Reade
J. P. Keating
Affiliation:
School of Mathematics, University of Bristol, Bristol BS8 1TW, UK and BRIMS, Hewlett-Packard Laboratories, Filton Road, Stoke Gifford, Bristol BS12 6QZ, UK
J. B. Reade
Affiliation:
Department of Mathematics, The University of Manchester, Manchester M13 9PL, UK
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The Abel and Cesàro summabilities of two alternating gap series are investigated. We prove that the series 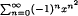is summable at x = 1 (in both senses), but that 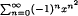is not. In 1907, Hardy obtained essentially the same result for the latter series; our proof is shorter and more elementary: we use the Poisson summation formula to derive an explicit estimate for the size of the oscillations as x → 1_. This represents an example of a general method for determining the Abel summability of similar series.

Keywords

summabilityPoisson summationgap series
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 43 , Issue 1 , February 2000 , pp. 95 - 101
Copyright
Copyright © Edinburgh Mathematical Society 2000

References

1.Abramowitz, M. and Stegun, I. A., Handbook of mathematical functions (Dover, New York, 1970).Google Scholar
2.Hardy, G. H., Q. J. Math. 38 (1907), 269–288.Google Scholar
3.Hardy, G. H., Divergent series (Oxford University Press, 1949).Google Scholar
4.Katznelson, Y., An introduction to harmonic analysis (Wiley, Jerusalem, 1968).Google Scholar