No CrossRef data available.
Published online by Cambridge University Press: 20 January 2009
In this paper we present three results about Arens regular bilinear operators. These are: (a). Let X, Y be two Banach spaces, K a compact Hausdorff space, µ a Borel measure on K and m: X × Y →ℂ a bounded bilinear operator. Then the bilinear operator  defined by
defined by  is regular iff m is regular, (b) Let (Xα), (Xα),(Zα) be three families of Banach spaces and let mα:Xα ×Yα→Zα, be a family of bilinear operators with supα∥mα∥<∞. Then the bilinear operator
is regular iff m is regular, (b) Let (Xα), (Xα),(Zα) be three families of Banach spaces and let mα:Xα ×Yα→Zα, be a family of bilinear operators with supα∥mα∥<∞. Then the bilinear operator 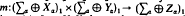 defined by
defined by  is regular iff each mα, is regular, (c) Let X, Y have the Dieudonné property and let m:X × Y→Z be a bounded bilinear operator with m(X×Y) separable and such that, for each z′ in ext Z′1, z′∘m is regular. Then m is regular. Several applications of these results are also given.
is regular iff each mα, is regular, (c) Let X, Y have the Dieudonné property and let m:X × Y→Z be a bounded bilinear operator with m(X×Y) separable and such that, for each z′ in ext Z′1, z′∘m is regular. Then m is regular. Several applications of these results are also given.