Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Berndt, Bruce C.
and
Yeap, Boon Pin
2002.
Explicit evaluations and reciprocity theorems for finite trigonometric sums.
Advances in Applied Mathematics,
Vol. 29,
Issue. 3,
p.
358.
Wang, Xin
and
Zheng, De-Yin
2007.
Summation formulae on trigonometric functions.
Journal of Mathematical Analysis and Applications,
Vol. 335,
Issue. 2,
p.
1020.
Hassan, H.A.
2008.
New trigonometric sums by sampling theorem.
Journal of Mathematical Analysis and Applications,
Vol. 339,
Issue. 2,
p.
811.
Annaby, M.H.
Hassan, H.A.
and
El-Haddad, O.H.
2009.
Perturbed Discrete Sturm-Liouville Problems and Associated Sampling Theorems.
Rocky Mountain Journal of Mathematics,
Vol. 39,
Issue. 6,
Annaby, M.H.
and
Asharabi, R.M.
2011.
Exact evaluations of finite trigonometric sums by sampling theorems.
Acta Mathematica Scientia,
Vol. 31,
Issue. 2,
p.
408.
Fonseca, Carlos M. da
Glasser, M. Lawrence
and
Kowalenko, Victor
2017.
Basic trigonometric power sums with applications.
The Ramanujan Journal,
Vol. 42,
Issue. 2,
p.
401.
Abd-Alla, M. Z.
Annaby, M. H.
Hassan, H. A.
and
Ayad, H. A.
2018.
Difference operators, Green's matrix, sampling theory and applications in signal processing.
Journal of Difference Equations and Applications,
Vol. 24,
Issue. 8,
p.
1318.
Chu, Wenchang
2018.
Reciprocal relations for trigonometric sums.
Rocky Mountain Journal of Mathematics,
Vol. 48,
Issue. 1,
Annaby, M.A.
and
Hassan, H.A.
2018.
Trigonometric sums by Hermite interpolations.
Applied Mathematics and Computation,
Vol. 330,
Issue. ,
p.
213.
He, Yuan
2020.
Explicit expressions for finite trigonometric sums.
Journal of Mathematical Analysis and Applications,
Vol. 484,
Issue. 1,
p.
123702.
Cvijović, Djurdje
2020.
Two general families of integer–valued polynomials associated with finite trigonometric sums.
Journal of Mathematical Analysis and Applications,
Vol. 488,
Issue. 1,
p.
124057.
Ejsmont, Wiktor
and
Lehner, Franz
2021.
The trace method for cotangent sums.
Journal of Combinatorial Theory, Series A,
Vol. 177,
Issue. ,
p.
105324.
Du, Zhibin
da Fonseca, Carlos M.
and
Kowalenko, Victor
2023.
Further developments of basic trigonometric power sums.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 117,
Issue. 3,
Chu, Wenchang
2023.
Trigonometric Sums and Riemann Zeta Function.
Mathematica Slovaca,
Vol. 73,
Issue. 3,
p.
595.
Allouche, Jean-Paul
and
Zeilberger, Doron
2023.
Human and automated approaches for finite trigonometric sums.
The Ramanujan Journal,
Vol. 62,
Issue. 1,
p.
189.
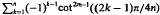 and
and  can be represented as integer-valued polynomials in n of degrees 2m – 1 and 2m, respectively. Properties of these polynomials are discussed, and recurrence relations for the coefficients are obtained. The proofs of the results depend on the representations of particular polynomials of degree n – 1 or less as their own Lagrange interpolation polynomials based on the zeros of the nth Chebyshev polynomial Tn(x) = cos(narccos x), -1≤x≤1.
can be represented as integer-valued polynomials in n of degrees 2m – 1 and 2m, respectively. Properties of these polynomials are discussed, and recurrence relations for the coefficients are obtained. The proofs of the results depend on the representations of particular polynomials of degree n – 1 or less as their own Lagrange interpolation polynomials based on the zeros of the nth Chebyshev polynomial Tn(x) = cos(narccos x), -1≤x≤1.

