Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-12-01T03:45:35.377Z Has data issue: false hasContentIssue false
  • English
  • Français

A singular perturbation problem for the Lavrent'ev-Bitsadze equation*

Published online by Cambridge University Press:  20 January 2009

R. J. Weinacht
R. J. Weinacht
Affiliation:
University of Delaware, Newark, Delaware 19711, USA Fachbereich MathematikTechnische Hochschule Darmstadt61 Darmstadt, West Germany
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this note we consider a singular perturbation problem for the equation

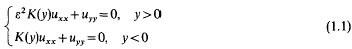
where K(y) = sgn y and. Ε is a small (positive) parameter. This equation for ε≠O is elliptic for y<0 and hyperbolic for y>0. Many of the results carry over to more difficult and interesting problems for equations of mixed type. The particularly simple model treated here permits the elimination of some complications in the analysis involving singular integral equations while preserving the main qualitative features of more general cases. For a special Tricomi-like problem for (1.1) we construct asymptotic expansions in ε, including boundary layer corrections, of the solution. A proof of uniform asymptotic validity of the lowest order terms is given.

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 26 , Issue 1 , February 1983 , pp. 49 - 66
Copyright
Copyright © Edinburgh Mathematical Society 1983

References

REFERENCES

1.Bitsadze, A. V., Equations of the Mixed Type (Pergamon Press, New York, 1964).Google Scholar
2.Eckhaus, W. and De Jager, E. M., Asymptotic solutions of singular perturbation problems forlinear differential equations of elliptic type, Arch. Rational Mech. and Anal. 23 (1966), 26–86.CrossRefGoogle Scholar
3.De Jager, E. M., Singular perturbations of hyperbolic type, Nieuw Archief voor Wiskunde (3), 12 (1975), 145–171.Google Scholar
4.Hsiao, G. C. and Weinacht, R. J., Singular perturbation problems for a class of singular partial differential equations, Lecture Notes in Mathematics 564 (1976), 249–258.CrossRefGoogle Scholar
5.Latta, G. E., Singular Perturbation Problems (Ph.D. Thesis, California Institute of Technology, 1951).Google Scholar
6.Keller, J. B., Perturbation Theory (Lecture Notes, Mathematics Department, Michigan StateUniversity, 1968).Google Scholar
7.Hopf, E., A remark on linear elliptic equations of second order, Proc. Amer. Math. Soc. 3 (1952), 791–793.CrossRefGoogle Scholar