Article contents
Radially symmetric solutions of a class of singular elliptic equations
Published online by Cambridge University Press: 20 January 2009
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
The boundary value problem
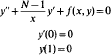
is studied with a view to obtaining the existence of positive solutions in C1([0, 1])∩C2((0, 1)). The function f is assumed to be singular in the second variable, with the singularity modeled after the special case f(x, y) = a(x)y−p, p>0.
This boundary value problem arises in the search of positive radially symmetric solutions to
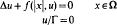
where Ω is the open unit ball in ℝN, centered at the origin, Γ is its boundary and |x| is the Euclidean norm of x.
- Type
- Research Article
- Information
- Copyright
- Copyright © Edinburgh Mathematical Society 1990
References
REFERENCES
1.Atkinson, F. V. and Peletier, L. A., Ground states of −Δu = f(u) and the Emden–Fowler equation, Arch. Rational Mech. Anal. 93 (1986), 103–107.CrossRefGoogle Scholar
2.Diaz, J. D., Morel, J. M. and Oswald, L., An elliptic equation with singular nonlinearity, Comm. Partial Differential Equations 12 (1987), 1333–1344.CrossRefGoogle Scholar
3.Erbe, L. and Schmitt, K., On radial solutions of some semilinear elliptic equations, Diff. and Int. Equations 1 (1988), 71–78.Google Scholar
4.Gatica, J. A., Oliker, V. and Waltman, P., Iterative procedures for nonlinear second order boundary value problems (Emory University preprint, 1987).Google Scholar
5.Gatica, J. A., Oliker, V. and Waltman, P., Singular nonlinear boundary value problems for second order differential equations, J. Differential Equations 79 (1989), 62–78.CrossRefGoogle Scholar
6.Gidas, B., Ni, Wei-Ming and Niremberg, L., Symmetry of positive solutions of nonlinear elliptic equations in ℝn, Adv. Math Suppl. Stud. 7A (1981), 369–402.Google Scholar
7.Nachman, A. and Callegari, A., A nonlinear boundary value problem in the theory of pseudoplastic fluids, SIAM J. Appl. Math. 38 (1980), 275–281.CrossRefGoogle Scholar
8.Nussbaum, R. D. and Stuart, C. A., A singular bifurcation problem, J. London Math. Soc. (2) 14 (1976), 31–38.CrossRefGoogle Scholar
9.Oliker, V. I., Near radially symmetric solutions of an inverse problem in geometric optics, Inverse Problems 3 (1987), 743–756.CrossRefGoogle Scholar
10.Stuart, C. A., Concave solutions of singular non-linear differential equations, Math. Z. 136 (1974), 117–135.CrossRefGoogle Scholar
11.Taliaferro, S., A nonlinear singular boundary value problem, Nonlinear Anal. 3 (1979), 897–904.CrossRefGoogle Scholar
12.Wong, J. S. W., On the generalized Emden–Fowler equation, SIAM Rev. 17 (1975), 339–360.CrossRefGoogle Scholar
- 17
- Cited by


