Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Cascales, B.
Orihuela, J.
and
Galán, M. Ruiz
2013.
Computational and Analytical Mathematics.
Vol. 50,
Issue. ,
p.
161.
Angosto, C.
Cascales, B.
and
Rodríguez, J.
2013.
Distances to spaces of measurable and integrable functions.
Mathematische Nachrichten,
Vol. 286,
Issue. 14-15,
p.
1424.
Kačena, Miroslav
Kalenda, Ondřej F.K.
and
Spurný, Jiří
2013.
Quantitative Dunford–Pettis property.
Advances in Mathematics,
Vol. 234,
Issue. ,
p.
488.
Bendová, Hana
2014.
Quantitative Grothendieck property.
Journal of Mathematical Analysis and Applications,
Vol. 412,
Issue. 2,
p.
1097.
Cascales, Bernardo
and
Orihuela, José
2014.
Recent Progress in General Topology III.
p.
93.
Gasparis, I.
2015.
An extension of James's compactness theorem.
Journal of Functional Analysis,
Vol. 268,
Issue. 1,
p.
194.
Bendová, Hana
Kalenda, Ondřej F.K.
and
Spurný, Jiří
2015.
Quantification of the Banach–Saks property.
Journal of Functional Analysis,
Vol. 268,
Issue. 7,
p.
1733.
Paúl, Pedro J.
2017.
A quantitative extension of a theorem of Valdivia on weak compactness.
Journal of Mathematical Analysis and Applications,
Vol. 456,
Issue. 2,
p.
1517.
Krulišová, Hana
2017.
Quantification of Pełczyński's property (V).
Mathematische Nachrichten,
Vol. 290,
Issue. 17-18,
p.
2909.
Cascales, B.
Orihuela, J.
and
Pérez, A.
2017.
One-sided James' compactness theorem.
Journal of Mathematical Analysis and Applications,
Vol. 445,
Issue. 2,
p.
1267.
Li, Lei
Chen, Dongyang
and
Chávez‐Domínguez, Javier Alejandro
2018.
Pełczyński's property () of order p and its quantification.
Mathematische Nachrichten,
Vol. 291,
Issue. 2-3,
p.
420.
Hamhalter, Jan
and
Kalenda, Ondřej F. K.
2019.
Measures of weak non-compactness in spaces of nuclear operators.
Mathematische Zeitschrift,
Vol. 292,
Issue. 1-2,
p.
453.
Angosto, Carlos
2019.
Distance to Spaces of Semicontinuous and Continuous Functions.
Journal of Function Spaces,
Vol. 2019,
Issue. ,
p.
1.
Hamhalter, Jan
Kalenda, Ondřej F.K.
Peralta, Antonio M.
and
Pfitzner, Hermann
2020.
Measures of weak non-compactness in preduals of von Neumann algebras and JBW⁎-triples.
Journal of Functional Analysis,
Vol. 278,
Issue. 1,
p.
108300.
García, G.
2021.
A quantitative version of the Kolmogorov–Riesz theorem.
Advances in Operator Theory,
Vol. 6,
Issue. 1,
Chen, Dongyang
Kania, Tomasz
and
Ruan, Yingbin
2022.
Quantifications of boundedly complete and shrinking bases.
Illinois Journal of Mathematics,
Vol. -1,
Issue. -1,
Chen, Dongyang
Kania, Tomasz
and
Ruan, Yingbin
2022.
$$(1+)$$-complemented, $$(1+)$$-isomorphic copies of $$L_{1}$$ in dual Banach spaces.
Archiv der Mathematik,
Vol. 119,
Issue. 5,
p.
495.
Chen, Dongyang
Kania, Tomasz
and
Ruan, Yingbin
2023.
Quantifying properties (K) and (μs$\mu ^{s}$).
Mathematische Nachrichten,
Vol. 296,
Issue. 3,
p.
996.
Silber, Zdeněk
2023.
Quantification of Banach-Saks properties of higher orders.
Journal of Mathematical Analysis and Applications,
Vol. 517,
Issue. 1,
p.
126590.
 ⊂ E** with x**(x*) = sup x*(C). A quantitative version of James's Compactness Theorem is proved using JaE and Ja, and in particular it yields the following result. Let C be a closed convex bounded subset of a Banach space E and r > 0. If there is an element
⊂ E** with x**(x*) = sup x*(C). A quantitative version of James's Compactness Theorem is proved using JaE and Ja, and in particular it yields the following result. Let C be a closed convex bounded subset of a Banach space E and r > 0. If there is an element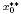 in
in whose distance to C is greater than r, then there is x* ∈ E* such that each x** ∈
whose distance to C is greater than r, then there is x* ∈ E* such that each x** ∈ at which sup x*(C) is attained has distance to E greater than ½r. We indeed establish that JaE and Ja are equivalent to other measures of weak non-compactness studied in the literature. We also collect particular cases and examples showing when the inequalities between the different measures of weak non-compactness can be equalities and when the inequalities are sharp.
at which sup x*(C) is attained has distance to E greater than ½r. We indeed establish that JaE and Ja are equivalent to other measures of weak non-compactness studied in the literature. We also collect particular cases and examples showing when the inequalities between the different measures of weak non-compactness can be equalities and when the inequalities are sharp.