Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-28T10:40:04.149Z Has data issue: false hasContentIssue false
  • English
  • Français

On The Uniformisation of Algebraic Curves of Genus 3

Published online by Cambridge University Press:  20 January 2009

M. Mursi
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

An algebraic equation

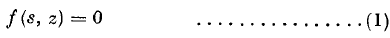
determines, in general, s as a many valued function of z. If s and z can be expressed as one valued functions of a third variable t, then t is called the uniformising variable. As Poincaré showed, s and z are automorphic functions of t.

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 2 , Issue 2 , June 1930 , pp. 102 - 107
Copyright
Copyright © Edinburgh Mathematical Society 1930

References

page 101 note 1 CfWhittaker, E. T., Phil. Trans., Royal Soc. (A) 192 (1899), 1.CrossRefGoogle Scholar

page 101 note 2 Journal London Math. Soc. 5 (1930).Google Scholar

page 101 note 3 Journal London Math. Soc. 4 (1929), 274.Google Scholar

page 106 note 1 This follows from the uniqueness of the group of the diflerential equation and alsofrom the symmetry of the positions of the contour in the plane.Google Scholar