On quadratic fields generated by the Shanks sequence

Florian Luca; Igor E. Shparlinski

doi:10.1017/S001309150700123X

On quadratic fields generated by the Shanks sequence

Part of: Multiplicative number theory Algebraic number theory: global fields Exponential sums and character sums Diophantine equations

Published online by Cambridge University Press: 23 September 2009

Florian Luca and

Igor E. Shparlinski

Show author details

Florian Luca: Affiliation:
Instituto de Matemáticas, Universidad Nacional Autonoma de México, CP 58089, Morelia, Michoacán, Mexico; Email: ([email protected])
Igor E. Shparlinski: Affiliation:
Department of Computing, Macquarie University, Sydney, NSW 2109, Australia; Email: ([email protected])

Article contents

Abstract

Rights & Permissions

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

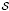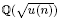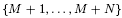Let u(n)=f(gn), where g > 1 is integer and f(X) ∈ ℤ[X] is non-constant and has no multiple roots. We use the theory of -unit equations as well as bounds for character sums to obtain a lower bound on the number of distinct fields among for n ∈ . Fields of this type include the Shanks fields and their generalizations.