No CrossRef data available.
Article contents
On functions with derivative of bounded variation: An analogue of Banach's indicatrix theorem
Published online by Cambridge University Press: 20 January 2009
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
A simple, but nice theorem of Banach states that the variation of a continuous function F:[a, b]→ ℝ is given by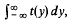 
where t(y) is defined as the number of x ∈ [a, b[ for which F(x)= y (see, e.g., [1], VIII.5, Th. 3). In this paper we essentially derive a similar representation for the variation of F′ which also yields a criterion for a function to be an integral of a function of bounded variation. The proof given here is quite elementary, though long and somewhat intriciate.
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 29 , Issue 1 , February 1986 , pp. 61 - 68
- Copyright
- Copyright © Edinburgh Mathematical Society 1986
References
REFERENCES
1.Natanson, I. P., Theory of Functions of a Real Variable (Frederick Ungar Publishing Co., New York, 1955).Google Scholar
2.Stadje, W., Bemerkung zu einem Satz von Akcoglu und Krengel, Studia Math. 81 (1985), 307–310.CrossRefGoogle Scholar
You have
Access