No CrossRef data available.
Article contents
On a certain class of Linear Substitutions with Common Invariants, and an Associated Substitution of Order Four
Published online by Cambridge University Press: 20 January 2009
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
The determinant
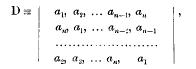
each row of wliich contains the same n elements in the same cyclic order, with ′a1 always in the leading diagonal, is the product of n linear factors, which we shall write as follows
where ρ is any primitive nth root of unity.
- Type
- Research Article
- Information
- Copyright
- Copyright © Edinburgh Mathematical Society 1912
References
page 54 note * See Scott, Determinants, p. 81.
page 55 note * Linear Substitutions and their Invariants, §2.Proc. Edin. Math. Soc., Vol. XXX.
page 55 note † Ibid. §9.
page 70 note * Since writing the above, I have found the following references to triangles in multiple perspective: —
Homologous Triangles, by Thomas Muir M.A., [Mess. Math. 2 (1873)]. “ Triply homologous” triangles are discussed, the vertices being taken in the same cyclic order throughout.
Perspective Dreiecke und Tetraeder, by Edmund Hess [Math. Annalen 28 (1887)]. Here sextuply perspective triangles are introduced, the standpoint being g-ometrical, and the application different from that of the present paper.
You have
Access