Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-28T06:12:12.415Z Has data issue: false hasContentIssue false
  • English
  • Français

A note on the correlation of classes

Published online by Cambridge University Press:  20 January 2009

J. M. Whittaker
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let R be a (1 — 1) relation between the members of two similar classes A, B1. It correlates the members of a subclass X of A to the members of a certain subclass Y of B1 and thus defines a relation ρ connecting X and Y. It is clear that ρ is a (1 – 1) relation and that it has the property (M). If X1ρ Y1, X2ρY2, then 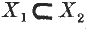implies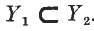

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 1 , Issue 1 , May 1927 , pp. 47 - 48
Copyright
Copyright © Edinburgh Mathematical Society 1927

References

page 47 note 1 There may be no L's, but this does not matter since the null class is counted as a subclass of A. It will be noticed that the proof depends only on the fact that ρ. σ are (1 – 1 ) relations with the property (M), so that the theorem is true for any relations with these properties. Thus it is not necessary that the members of X should be in (1 – 1) relation with those of Y, nor that those subclasses of B to which the subclasses of A are correlated by ρ should be all the subclasses of a certain part B1 of B.

page 48 note 1 i.e. if a, b are cardinal numbers, a ≤ b and b ≤ a together imply a = b.