Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-26T00:40:59.244Z Has data issue: false hasContentIssue false
  • English
  • Français

A Note on Griffith Cracks†

Published online by Cambridge University Press:  20 January 2009

M. Lowengrub
M. Lowengrub
Affiliation:
Wesleyan and Duke Universities
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The problem of determining the distribution of stress in the neighbourhood of a Griffith crack, denned by ∣x∣ ≦ 1, y = 0, which is subject to an internal pressure varying along the length of the crack has been solved by Sneddon and Elliott (3) and by England and Green (1). In the former paper a Fourier cosine method is used to arrive at a solution while in the latter paper the problem is reduced to an Abel integral equation by making use of integral representations of the complex potentials given in Green and Zerna (2). Neither paper deals with the calculation of the stress intensity factor

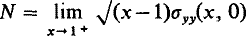
which is extremely important to workers in fracture mechanics.

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 15 , Issue 2 , December 1966 , pp. 131 - 134
Copyright
Copyright © Edinburgh Mathematical Society 1966

References

REFERENCES

(1) England, A. H. and Green, A. E., Some two-dimensional punch and crack problems in classical elasticity, Proc. Cambridge Phil. Soc. 59 (1963), 489.CrossRefGoogle Scholar
(2) Green, A. E. and Zerna, W., Theoretical Elasticity (Oxford University Press, 1954).Google Scholar
(3) Sneddon, I. N. and Elliott, H. A., The opening of a Griffith crack under internal pressure, Quart. Appl. Math. 4 (1946), 262.CrossRefGoogle Scholar
(4) Sneddon, I. N., The elementary solution of dual integral equations, Proc. Glasgow Math. Assoc. 4 (1960), 108.CrossRefGoogle Scholar
(5) Sneddon, I. N., Fourier Transforms (McGraw-Hill; New York, 1951).Google Scholar