Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-26T00:54:02.006Z Has data issue: false hasContentIssue false
  • English
  • Français

Infinite quasi-injective groups

Published online by Cambridge University Press:  20 January 2009

M. J. Tomkinson
M. J. Tomkinson
Affiliation:
Department of Mathematics, University of Glasgow, Glasgow G12 8QW
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

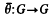A group G is said to be quasi-injective if, for each subgroup H of G and homomorphism θ:H→G, there is an endomorphism such that 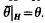. It is of course well known that the category of groups does not possess non-trivial injective objects and so we consider groups satisfying the weaker condition of quasi-injectivity.

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 31 , Issue 2 , June 1988 , pp. 249 - 259
Copyright
Copyright © Edinburgh Mathematical Society 1988

References

REFERENCES

1.Bertholf, D. and Walls, G., Finite quasi-injective groups, Glasgow Math. J. 20 (1979), 29–33.CrossRefGoogle Scholar
2.Fuchs, L., Infinite Abelian Groups I (Academic Press, 1970).Google Scholar
3.Huppert, B., Endliche Gruppen I (Grundlehren der math. Wiss. 134, Springer, 1967).CrossRefGoogle Scholar
4.Neumann, B. H., Groups with finite classes of conjugate subgroups, Math. Z. 63 (1955), 76–96.CrossRefGoogle Scholar
5.Oušanskiĭ, A. Yu., An infinite group whose subgroups are all of prime order, Izv. Akad. Nauk SSSR Ser. Mat. 44 (1980), 309–321.Google Scholar
6.Robinson, D. J. S., Groups in which normality is a transitive relation, Proc. Cambridge Philos. Soc. 60 (1964), 21–38.CrossRefGoogle Scholar
7.Robinson, D. J. S., Finiteness Conditions and Generalized Soluble Groups, Part I (Ergebnisse der Math. 62, Springer, 1972).Google Scholar