Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
LANDSTAD, MAGNUS B.
and
LARSEN, NADIA S.
2009.
GENERALIZED HECKE ALGEBRAS AND C*-COMPLETIONS.
International Journal of Mathematics,
Vol. 20,
Issue. 01,
p.
45.
Amini, Massoud
2011.
C*-algebras of generalized Hecke pairs.
Mathematica Slovaca,
Vol. 61,
Issue. 4,
p.
645.
Kaliszewski, S.
Landstad, Magnus B.
and
Quigg, John
2012.
A crossed-product approach to the Cuntz–Li algebras.
Proceedings of the Edinburgh Mathematical Society,
Vol. 55,
Issue. 2,
p.
429.
Amini, Massoud
2012.
Crossed product of c*-algebras by hypergroups via group coactions.
Mathematica Slovaca,
Vol. 62,
Issue. 3,
p.
587.
Palma, Rui
2013.
Operator Algebra and Dynamics.
Vol. 58,
Issue. ,
p.
253.
Palma, Rui
2013.
On envelopingC⁎-algebras of Hecke algebras.
Journal of Functional Analysis,
Vol. 264,
Issue. 12,
p.
2704.
LARSEN, NADIA S.
and
PALMA, RUI
2016.
Positive definite *-spherical functions, property (T) and C*-completions of Gelfand pairs.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 160,
Issue. 1,
p.
77.
AOI, Hisashi
and
YAMANOUCHI, Takehiko
2017.
Hecke pairs of ergodic discrete measured equivalence relations and the Schlichting completion.
Journal of the Mathematical Society of Japan,
Vol. 69,
Issue. 4,
Hendel, Yotam I
2021.
On Twisted Gelfand Pairs Through Commutativity of a Hecke Algebra.
International Mathematics Research Notices,
Vol. 2021,
Issue. 12,
p.
9203.
Džambić, Amir
and
González-Diez, Gabino
2021.
Galois action on Fuchsian surface groups and their solenoids.
Forum Mathematicum ,
Vol. 33,
Issue. 2,
p.
375.
Oppelmayer, Hanna
2021.
Boundary entropy spectra as finite subsums.
Stochastics and Dynamics,
Vol. 21,
Issue. 06,
p.
2150038.
Arimoto, Ryoya
2022.
On the type of the von Neumann algebra of an open subgroup of the Neretin group.
Proceedings of the American Mathematical Society, Series B,
Vol. 9,
Issue. 29,
p.
311.
Valvekens, Matthias
2022.
L2-Betti Numbers ofC*-Tensor Categories Associated with Totally Disconnected Groups.
International Mathematics Research Notices,
Vol. 2022,
Issue. 14,
p.
10704.
Skalski, Adam
Vergnioux, Roland
and
Voigt, Christian
2023.
Hecke algebras and the Schlichting completion for discrete quantum groups.
Journal of the London Mathematical Society,
Vol. 107,
Issue. 3,
p.
843.
De Commer, K.
2025.
Induction for representations of coideal doubles, with an application to quantum SL(2,R).
Indagationes Mathematicae,
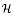 of a Hecke pair (G, H) is studied using the Schlichting completion (Ḡ,
of a Hecke pair (G, H) is studied using the Schlichting completion (Ḡ, 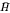 ), which is a Hecke pair whose Hecke algebra is isomorphic to
), which is a Hecke pair whose Hecke algebra is isomorphic to 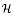 and which is topologized so that
and which is topologized so that 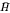 is a compact open subgroup of Ḡ. In particular, the representation theory and C*-completions of
is a compact open subgroup of Ḡ. In particular, the representation theory and C*-completions of 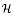 are addressed in terms of the projection
are addressed in terms of the projection  using both Fell's and Rieffel's imprimitivity theorems and the identity
using both Fell's and Rieffel's imprimitivity theorems and the identity 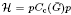 . An extended analysis of the case where H is contained in a normal subgroup of G (and in particular the case where G is a semi-direct product) is carried out, and several specific examples are analysed using this approach.
. An extended analysis of the case where H is contained in a normal subgroup of G (and in particular the case where G is a semi-direct product) is carried out, and several specific examples are analysed using this approach.