No CrossRef data available.
Article contents
A Generalisation of a Theorem of Mercer
Published online by Cambridge University Press: 20 January 2009
Extract
§ 1. It is well known that, if  , the convergence of sn to a limit implies the convergence of tn to the same limit. The converse theorem, that the convergence of tn implies the convergence of sn, is false. Mercer1 proved, however, that if
, the convergence of sn to a limit implies the convergence of tn to the same limit. The converse theorem, that the convergence of tn implies the convergence of sn, is false. Mercer1 proved, however, that if 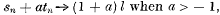 , then both sn and tn tend to l. This theorem has recently been extended in various directions.2 In the present note the case of Abel limits is considered.
, then both sn and tn tend to l. This theorem has recently been extended in various directions.2 In the present note the case of Abel limits is considered.
- Type
- Research Article
- Information
- Copyright
- Copyright © Edinburgh Mathematical Society 1930
References
page 108 note 1 Proc. London Math. Soc., (2), 5, (1907), 206–224.Google Scholar
page 108 note 2 Cf. Vijayaraghavan, Journal London Math. Soc., 3, (1928), 130–134, (who gives references to previous work on the subject); Copson and Ferrar, ibid., 4, (1929), 258–264 ; 5 (1930), 21–27.
page 108 note 3 See, for example, Knopp, Infinite Series, (1928), 498et seq.Google Scholar
page 110 note 1 Journal London Math. Soc., 4 (1929), 258–264; Theorem IV .Google Scholar


