Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-25T04:33:19.609Z Has data issue: false hasContentIssue false
  • English
  • Français

Extension and averaging operators for finite fields

Part of: Finite fields and commutative rings (number-theoretic aspects) Harmonic analysis in several variables

Published online by Cambridge University Press:  30 April 2013

Doowon Koh  and
Chun-Yen Shen
Doowon Koh
Affiliation:
Department of Mathematics, Chungbuk National University, Cheongju City, Chungbuk-Do 361-763, Republic of Korea ([email protected])
Chun-Yen Shen
Affiliation:
Department of Mathematics and Statistics, McMaster University, Hamilton L8S 4K1, Canada ([email protected])
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper we study Lp−Lr estimates of both the extension operator and the averaging operator associated with the algebraic variety S = {x ∈ : Q(x) = 0}, where Q(x) is a non-degenerate quadratic form over the finite field with q elements. We show that the Fourier decay estimate on S is good enough to establish the sharp averaging estimates in odd dimensions. In addition, the Fourier decay estimate enables us to simply extend the sharp L2−L4 conical extension result in 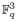, due to Mockenhaupt and Tao, to the L2−L2(d+1)/(d−1) estimate in all odd dimensions d ≥ 3. We also establish a sharp estimate of the mapping properties of the average operators in the case when the variety S in even dimensions d ≥ 4 contains a d/2-dimensional subspace.

Keywords

extension problemaveraging operatorfinite field

MSC classification

Secondary: 42B05: Fourier series and coefficients 11T24: Other character sums and Gauss sums
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 56 , Issue 2 , June 2013 , pp. 599 - 614
Copyright
Copyright © Edinburgh Mathematical Society 2013

References

1.Bourgain, J., On the restriction and multiplier problem in ℝ3, Lecture Notes in Mathematics, Volume 1469 (Springer, 1991).Google Scholar
2.Carbery, A., Stones, B. and Wright, J., Averages in vector spaces over finite fields, Math. Proc. Camb. Phil. Soc. 144(13) (2008), 13–27.CrossRefGoogle Scholar
3.De Carli, L. and Iosevich, A., Some sharp restriction theorems for homogeneous manifolds, J. Fourier Analysis Applic. 1(4) (1998), 105–128.CrossRefGoogle Scholar
4.Iosevich, A. and Sawyer, E., Sharp Lp–Lq estimates for a class of averaging operators, Annales Inst. Fourier 46(5) (1996), 1359–1384.CrossRefGoogle Scholar
5.Iwaniec, H. and Kowalski, E., Analytic number theory, Collo quium Publications, Volume 53 (American Mathematical Society, Providence, RI, 2004).Google Scholar
6.Lidl, R. and Niederreiter, H., Finite fields (Cambridge University Press, 1997).Google Scholar
7.Littman, W., Lp–Lq estimates for singular integral operators, Proc. Symp. Pure Math. 23 (1973), 479–481.CrossRefGoogle Scholar
8.Mockenhaupt, G. and Tao, T., Restriction and Kakeya phenomena for finite fields, Duke Math. J. 121(1) (2004), 35–74.CrossRefGoogle Scholar
9.Stein, E. M., Lpboundedness of certain convolution operators, Bull. Am. Math. Soc. 77 (1971), 404–405.CrossRefGoogle Scholar
10.Stein, E. M., Harmonic analysis (Princeton University Press, 1993).Google Scholar
11.Strichartz, R., Convolutions with kernels having singularities on the sphere, Trans. Am. Math. Soc. 148 (1970), 461–471.CrossRefGoogle Scholar
12.Tao, T., Recent progress on the restriction conjecture, in Fourier analysis and convexity (ed. Brandolini, L., Colzani, L., Iosevich, A. and Travaglini, G.), Applied and Numerical Harmonic Analysis, pp. 217–243 (Birkhäuser, Boston, MA, 2004).CrossRefGoogle Scholar
13.Wolff, T., A sharp bilinear cone restriction estimate, Annals Math. 153 (2001), 661–698.CrossRefGoogle Scholar