No CrossRef data available.
 $K3$ surfaces
$K3$ surfacesPublished online by Cambridge University Press: 08 August 2023
We consider three different families of Vafa–Witten invariants of 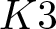 $K3$ surfaces. In each case, the partition function that encodes the Vafa–Witten invariants is given by combinations of twisted Dedekind η-functions. By utilizing known properties of these η-functions, we obtain exact formulae for each of the invariants and prove that they asymptotically satisfy all higher-order Turán inequalities.
$K3$ surfaces. In each case, the partition function that encodes the Vafa–Witten invariants is given by combinations of twisted Dedekind η-functions. By utilizing known properties of these η-functions, we obtain exact formulae for each of the invariants and prove that they asymptotically satisfy all higher-order Turán inequalities.