Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-28T07:11:18.305Z Has data issue: false hasContentIssue false
  • English
  • Français

Convexity and generalized Bernstein polynomials

Published online by Cambridge University Press:  20 January 2009

Tim N. T. Goodman ,
Halil Oruç  and
George M. Phillips
Tim N. T. Goodman
Affiliation:
Department of Mathematics and Computer Science, University of Dundee, Dundee, DD1 4HN
Halil Oruç
Affiliation:
Department of Mathematics and Computer Science, University of Dundee, Dundee, DD1 4HN
George M. Phillips
Affiliation:
Mathematical Institute, University of St Andrews, North Haugh St Andrews, Fife, K Y 16 9SS
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

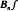In a recent generalization of the Bernstein polynomials, the approximated function f is evaluated at points spaced at intervals which are in geometric progression on [0, 1], instead of at equally spaced points. For each positive integer n, this replaces the single polynomial Bnf by a one-parameter family of polynomials , where 0 < q ≤ 1. This paper summarizes briefly the previously known results concerning these generalized Bernstein polynomials and gives new results concerning 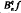when f is a monomial. The main results of the paper are obtained by using the concept of total positivity. It is shown that if f is increasing then 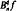is increasing, and if f is convex then 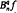is convex, generalizing well known results when q = 1. It is also shown that if f is convex then, for any positive integer n This supplements the well known classical result that 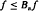when f is convex.

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 42 , Issue 1 , February 1999 , pp. 179 - 190
Copyright
Copyright © Edinburgh Mathematical Society 1999

References

REFERENCES

1.Goodman, T. N. T., Total positivity and the shape of curves, in Total Positivity and its Applications (Gasca, M., Micchelli, C. A. (eds.), Kluwer, Dordrecht, 1996), 157–186.Google Scholar
2.Lee, S. L. and Phillips, G. M., Polynomial interpolation at points of a geometric mesh on a triangle, Proc. Roy. Soc. Edinburgh 108A (1988), 75–87.CrossRefGoogle Scholar
3.Oruç, H. and Phillips, G. M., A generalization of the Bernstein polynomials, Proc. Edinburgh Math. Soc., to appear.Google Scholar
4.Phillips, G. M., Bernstein polynomials based on the q-integers, Ann. Numer. Math. 4 (1997), 511–518.Google Scholar
5.Phillips, G. M., A de Casteljau algorithm for generalized Bernstein polynomials, BIT 36 (1996), 232–236.Google Scholar
6.Schoenberg, I. J., On polynomial interpolation at the points of a geometric progression, Proc. Roy. Soc. Edinburgh 90A (1981), 195–207.Google Scholar