Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Helliwell, J. B.
1961.
A Flow Pattern at High Subsonic Speeds Past a Wedge at Incidence in a Free Stream and a Choked Channel.
Journal of Mathematics and Physics,
Vol. 40,
Issue. 1-4,
p.
1.
Helliwell, J. B.
1961.
Unsymmetrical flow patterns past a finite wedge profile in a High subsonic stream.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 57,
Issue. 2,
p.
401.
Bartlett, C. C.
and
Noble, B.
1961.
A Derivation of Certain Variational Principles for Mixed Boundary Value Problems in Potential Theory.
Proceedings of the Edinburgh Mathematical Society,
Vol. 12,
Issue. 3,
p.
113.
Erdogan, F.
and
Bahar, L. Y.
1964.
On the Solution of Simultaneous Dual Integral Equations.
Journal of the Society for Industrial and Applied Mathematics,
Vol. 12,
Issue. 3,
p.
666.
Folias, Efthymios S.
1965.
The Stresses in a Cracked Spherical Shell.
Journal of Mathematics and Physics,
Vol. 44,
Issue. 1-4,
p.
164.
1969.
The theory of absolute surface shear viscosity. I.
Proceedings of the Royal Society of London. A. Mathematical and Physical Sciences,
Vol. 310,
Issue. 1502,
p.
359.
Leppington, Frank
and
Levine, Harold
1970.
On the capacity of the circular disc condenser at small separation.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 68,
Issue. 1,
p.
235.
Folias, E. S.
1977.
Plates and shells with cracks.
p.
117.
Choo, S.C.
Leong, M.S.
and
Tan, L.S.
1981.
Spreading resistance calculations by the variational method.
Solid-State Electronics,
Vol. 24,
Issue. 6,
p.
557.
Leong, M.S.
Choo, S.C.
Tan, L.S.
and
Goh, T.L.
1988.
Contact resistance calculations based on a variational method.
Solid-State Electronics,
Vol. 31,
Issue. 7,
p.
1187.
PIERCE, ALLAN D.
1993.
Vol. 22,
Issue. ,
p.
195.
Rao, T V
2005.
Capacity of the circular plate condenser: analytical solutions for large gaps between the plates.
Journal of Physics A: Mathematical and General,
Vol. 38,
Issue. 46,
p.
10037.
Yang, Mimi X.
and
Yang, Fuqian
2015.
Capacitance analysis of a circular disk on a dielectric layer.
Journal of Electrostatics,
Vol. 76,
Issue. ,
p.
48.


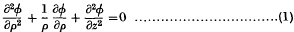
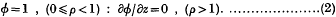
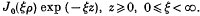 .
.