Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-29T21:11:32.886Z Has data issue: false hasContentIssue false
  • English
  • Français

Positive solutions for a degenerate Kirchhoff problem

Part of: Equations of mathematical physics and other areas of application Elliptic equations and systems

Published online by Cambridge University Press:  19 August 2021

David Arcoya ,
João R. Santos Júnior  and
Antonio Suárez
David Arcoya
Affiliation:
Dpto. de Análisis Matemático, Univ. de Granada, Granada18071, Spain ([email protected])
João R. Santos Júnior
Affiliation:
Faculdade de Matemática, Instituto de Ciências Exatas e Naturais, Univ. Federal do Pará, Avda. Augusto Corrêa 01, Belém, PA66075-110, Brazil ([email protected])
Antonio Suárez
Affiliation:
Dpto. de Ecuaciones Diferenciales y Análisis Numérico, Facultad de Matemáticas, Sevilla, Spain ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

By assuming that the Kirchhoff term has $K$ degeneracy points and other appropriated conditions, we have proved the existence of at least $K$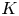 positive solutions other than those obtained in Santos Júnior and Siciliano [Positive solutions for a Kirchhoff problem with vanishing nonlocal term, J. Differ. Equ. 265 (2018), 2034–2043], which also have ordered $H_{0}^{1}(\Omega )$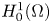-norms. A concentration phenomena of these solutions is verified as a parameter related to the area of a region under the graph of the reaction term goes to zero.

Keywords

degenerate Kirchhoff problemvariational methodsconcentration phenomena

MSC classification

Primary: 35J20: Variational methods for second-order elliptic equations 35J25: Boundary value problems for second-order elliptic equations
Secondary: 35Q74: PDEs in connection with mechanics of deformable solids
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 64 , Issue 3 , August 2021 , pp. 675 - 688
Check for updates
Copyright
Copyright © The Author(s), 2021. Published by Cambridge University Press on Behalf of The Edinburgh Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alves, C. O., Corrêa, F. J. S. A. and Ma, T. F., Positive solutions for a quasilinear elliptic equation of Kirchhoff type, Comput. Math. Appl. 49 (2005), 85–93.CrossRefGoogle Scholar
Ambrosetti, A. and Arcoya, D., Remarks on non homogeneous elliptic Kirchhoff equations, Nonlinear Differ. Equ. Appl. 23 (2016), Art. 57.CrossRefGoogle Scholar
Ambrosetti, A. and Arcoya, D., Positive solutions of elliptic Kirchhoff equations, Adv. Nonlinear Stud. 17 (2017), 3–16.CrossRefGoogle Scholar
Ambrosetti, A. and Prodi, G., A primer of nonlinear analysis (Cambridge University Press, Cambridge, 1993).Google Scholar
Brown, K. J. and Budin, H., On the existence of positive solutions for a class of semilinear elliptic boundary value problems, SIAM J. Math. Anal. 10 (1979), 875–883.CrossRefGoogle Scholar
Clément, P. and Sweers, G., Existence and multiplicity results for a semilinear eigenvalue problem, Annali della Scuola Normale Superiore di Pisa, (4) 14 (1987), 97–121.Google Scholar
He, X. and Zou, W., Infinitely many positive solutions for Kirchhoff-type problems, Nonlinear Anal. 70 (2009), 1407–1414.CrossRefGoogle Scholar
Hess, P., On multiple positive solutions of nonlinear elliptic eigenvalue problems, Comm. Partial Diff. Equ. 6 (1981), 951–961.CrossRefGoogle Scholar
Kirchhoff, G., Mechanik (Teubner, Leipzig, 1883).Google Scholar
Ma, T. F. and Muñoz Rivera, J. E., Positive solutions for a nonlinear nonlocal elliptic transmission problem, Appl. Math. Lett. 16 (2003), 243–248.CrossRefGoogle Scholar
Perera, K. and Zhang, Z., Nontrivial solutions of Kirchhoff-type problems via the Yang index, J. Differ. Equ. 221 (2006), 246–255.CrossRefGoogle Scholar
Perera, K. and Zhang, Z., Sign changing solutions of Kirchhoff type problems via invariant sets of descent flow, J. Math. Anal. Appl. 317 (2006), 456–463.Google Scholar
Santos Júnior, J. R. and Siciliano, G., Positive solutions for a Kirchhoff problem with vanishing nonlocal term, J. Differ. Equ. 265 (2018), 2034–2043.CrossRefGoogle Scholar