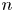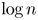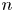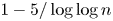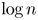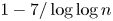Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Liu, Hailin
Lu, Longzhi
and
Zhong, Liping
2024.
On the proportion of elements of order a product of two primes in finite symmetric groups.
AIMS Mathematics,
Vol. 9,
Issue. 9,
p.
24394.
陈, 守双
2024.
Proportion of Elements of Prime Square Order in a Finite Symmetric Group.
Pure Mathematics,
Vol. 14,
Issue. 06,
p.
373.
Liu, Hailin
and
Zhong, Liping
2025.
On the proportion of elements of order $2p$ in finite symmetric groups.
Hacettepe Journal of Mathematics and Statistics,
Vol. 54,
Issue. 1,
p.
173.