Article contents
Dense subalgebras of purely infinite simple groupoid C*-algebras
Published online by Cambridge University Press: 30 March 2020
Abstract
A simple Steinberg algebra associated to an ample Hausdorff groupoid G is algebraically purely infinite if and only if the characteristic functions of compact open subsets of the unit space are infinite idempotents. If a simple Steinberg algebra is algebraically purely infinite, then the reduced groupoid 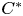 $C^*$-algebra
$C^*$-algebra  $C^*_r(G)$ is simple and purely infinite. But the Steinberg algebra seems too small for the converse to hold. For this purpose we introduce an intermediate *-algebra B(G) constructed using corners
$C^*_r(G)$ is simple and purely infinite. But the Steinberg algebra seems too small for the converse to hold. For this purpose we introduce an intermediate *-algebra B(G) constructed using corners 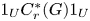 $1_U C^*_r(G) 1_U$ for all compact open subsets U of the unit space of the groupoid. We then show that if G is minimal and effective, then B(G) is algebraically properly infinite if and only if
$1_U C^*_r(G) 1_U$ for all compact open subsets U of the unit space of the groupoid. We then show that if G is minimal and effective, then B(G) is algebraically properly infinite if and only if 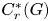 $C^*_r(G)$ is purely infinite simple. We apply our results to the algebras of higher-rank graphs.
$C^*_r(G)$ is purely infinite simple. We apply our results to the algebras of higher-rank graphs.
Keywords
MSC classification
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 63 , Issue 3 , August 2020 , pp. 609 - 629
- Copyright
- Copyright © Edinburgh Mathematical Society 2020
References
- 4
- Cited by



