No CrossRef data available.
Article contents
§2. Circumcentre
Published online by Cambridge University Press: 20 January 2009
Abstract
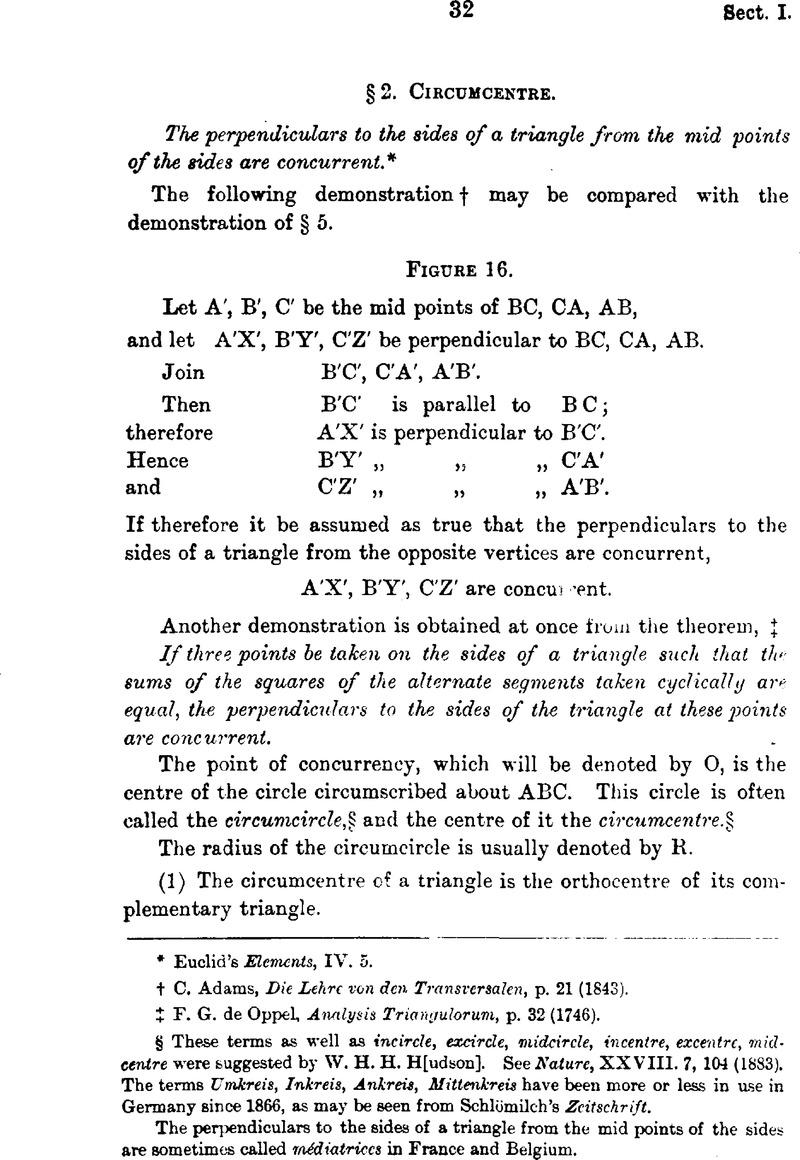
- Type
- Other
- Information
- Copyright
- Copyright © Edinburgh Mathematical Society 1883
References
* Euclid's, Elements, IV. 5.Google Scholar
† Adams, C., Die Lehrc von den Transrersalen, p. 21 (1843).Google Scholar
‡ de Oppel, F. G., Aimlyais Trianijulorum, p. 32 (1746).Google Scholar
§ These terms as well as incircle, excirde, midcircle, incentre, excentrc, midcentre were suggested by W. H. H. H[udson]. See Nature, XXVIII. 7, 104 (1883). The terms Umkreis, Inkreis, Ankreu, Mitienkreis have been more or less in use in Germany since 1866, as may be seen from Schlomilch's Zeitschrift.Google Scholar
The perpendiculars to the sides of a triangle from the mid points of the sides are sometimes called méediatrices in France and Belgium.
* Ladies' Diary for 1785.
† Given without proof in the Ladies' Diary for 1759.
‡ Rev. Wolstenholme, J. in the Educational Times, XXIX., 273 (1877).Google Scholar Four solutions are given in Mathematical Questions from the Educational Times, XXVII, 63–66 (1877); the one in the text is the last.Google Scholar
* Todhunter's, Plane Trigonouetry, Chap. XVI., Ex. 41 (1859).Google Scholar
† Both (7) and (8) are due to Mr E. M. Lingley, who applies the first of them to the problem of finding the triangle of minimum perimeter inscribed in a given triangle, and to the determination of the trilinear co-ordinates of the Broeard points. See Sixteenth General Report of the Astociatimi for the Improvemtnt of Geometrical Teaching, pp. 34–5 (1890).
* Jacobi, C. F. A., De Triangulorum Kecttiineorum Proprietatibus, p. 56 (1825).Google Scholar
† The first part of the theorem is given by Jacobi, p. 56.
* Jacobi does not state this property, but from the way in which he letter the figures of theorems (11) and (12) it is probable that he knew it. The property is explicitly stated, along with some others, by Mr Lemoine in his paper read at the Lyons meeting (1873) of the Association Franmise pour l'arancemcnt des Seiences.
† Rochat in Gergonne's, Annales, II. 29 (1811). To him also are due (17) and (19).Google Scholar


