Hostname: page-component-745bb68f8f-v2bm5 Total loading time: 0 Render date: 2025-01-11T09:45:22.688Z Has data issue: false hasContentIssue false
  • English
  • Français

Thick sets and quasisymmetric maps

Published online by Cambridge University Press:  22 January 2016

Jussi Väisälä ,
Matti Vuorinen  and
Hans Wallin
Jussi Väisälä
Affiliation:
Mathematiikan laitos, Helsingin yliopisto, Hallituskatu 15, FIN-00100 Helsinki, Finland
Matti Vuorinen
Affiliation:
Mathematiikan laitos, Helsingin yliopisto, Hallituskatu 15, FIN-00100 Helsinki, Finland
Hans Wallin
Affiliation:
Matematiska institutionen, Umeå universitet, S-90187 Umeå, Sweden
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

1.1. Thickness. Let E be a real inner product space. For a finite sequence of points a0, . . . ,ak in E we let a0. . . ,ak denote the convex hull of the set {a0, . . . , ak}. If these points are affinely independent, the set Δ = a0. . .ak is a k-simplex with vertices a0. . . ,ak. It has a well-defined k-volume written as mk(Δ) or briefly as m(Δ). We are interested in sets A ⊂ E which are “nowhere too flat in dimension k”. More precisely, suppose that A ⊂ E, q > 0 and that k: is a positive integer. 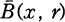We let denote the closed ball with center x and radius r. We say that A is (q, k)-thick if for each x ∈ A and r> 0 such that A\ 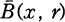≠ 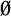there is a k-simplex Δ with vertices in A ∩ 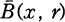such that mk(Δ) ≥ qr.

Type
Research Article
Information
Nagoya Mathematical Journal , Volume 135 , September 1994 , pp. 121 - 148
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 1994

References

[AG] Agard, S. and Gehring, F. W., Angles and quasiconformal mappings, Proc. London Math. Soc, 14A (1965), 1–21.Google Scholar
[AW] Anderson, G. D., Vamanamurthy, M. K. and Vuorinen, M., Inequalities for quasiconformal maps in space, Pacific Math. J., 160 (1993), 1–18.Google Scholar
[Be] Berger, M., Geometry I, Springer-Verlag, 1987.Google Scholar
[B1] Blumenthal, L. M., Theory and applications of distance geometry, Oxford at the Clarendon Press, 1953.Google Scholar
[Ha] Hadwiger, H., Vorlesungen über Inhalt, Oberfläche und Isoperimetrie, Springer-Verlag, 1957.CrossRefGoogle Scholar
[JW] Jonsson, A. and Wallin, H., Function spaces on subsets of Rn , Harwood Acad. Publ., 1984.Google Scholar
[MV] Mattila, P. and Vuorinen, M., Linear approximation property, Minkowski dimension and quasiconformal spheres, J. London Math. Soc, (2) 42 (1990), 249–269.Google Scholar
[NV] Näkki, R. and Väisälä, J., John disks, Exposition. Math., 9 (1991), 3–43.Google Scholar
[Pa] Partanen, J., Invariance theorems for the bilipschitz and quasisymmetric extension properties, Ann. Acad. Sci. Fenn. Ser. A I Math. Diss. 80 (1990), 1–40.Google Scholar
[Ri] Rickman, S., Characterization of quasiconformal arcs, Ann. Acad. Sci. Fenn. Ser. A I Math., 395 (1966), 1–30.Google Scholar
[Ro] Rohde, S., On conformal welding and quasicircles, Michigan Math. J., 38 (1991), 111–116.Google Scholar
[So] Sommerville, D. M. Y., An introduction to the geometry of n dimensions, Dover Publications, 1958.Google Scholar
[TV1] Tukia, P. and Väisälä, J., Quasisymmetric embeddings of metric spaces, Ann. Acad. Sei. Fenn. Ser. A I Math., 5 (1980), 97–114.Google Scholar
[TV2] Tukia, P., Extension of embeddings close to isometries or similarities, Ann. Acad. Sci. Fenn. Ser. A I Math., 9 (1984), 153–175.Google Scholar
[Vä1] Väisälä, J., Lectures on w-dimensional quasiconformal mappings, Lecture Notes in Mathematics, 229, Springer-Verlag, 1971.CrossRefGoogle Scholar
[Vä2] Väisälä, J., Quasimöbius maps, J. Analyse Math., 44, (1984/85), 218–234.Google Scholar
[Vä3] Väisälä, J., Bilipschitz and quasisymmetric extension properties, Ann. Acad. Sci. Fenn. Ser. A I Math., 11 (1986), 239–274.Google Scholar
[Vä4] Väisälä, J., Quasiconformal maps of cylindrical domains, Acta Math., 162 (1989), 201–225.Google Scholar
[VV] Vamanamurthy, M. K. and Vuorinen, M., Functional inequalities, Jacobi products, and quasiconformal maps, Illinois J. Math., 38 (1994), 394–419.Google Scholar
[Vu] Vuorinen, M., Quadruples and spatial quasiconformal mappings, Math. Z., 205 (1990), 617–628.Google Scholar
[WW] Wallin, H. and Wingren, P., Dimension and geometry of sets defined by polynomial inequalities, J. Approx. Theory 69 (1992), 231–249.Google Scholar