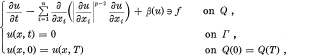Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kawohl, Bernhard
1981.
On nonlinear parabolic equations with abruptly changing nonlinear boundary conditions.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 5,
Issue. 10,
p.
1141.
Ôtani, Mitsuharu
1982.
Nonmonotone perturbations for nonlinear parabolic equations associated with subdifferential operators, Cauchy problems.
Journal of Differential Equations,
Vol. 46,
Issue. 2,
p.
268.
Okochi, Hiroko
1984.
Asymptotic behavior of solutions to certain nonlinear parabolic evolution equations.
Hiroshima Mathematical Journal,
Vol. 14,
Issue. 2,
Ôtani, Mitsuharu
1984.
Nonmonotone perturbations for nonlinear parabolic equations associated with subdifferential operators, periodic problems.
Journal of Differential Equations,
Vol. 54,
Issue. 2,
p.
248.
Okochi, Hiroko
1990.
On the existence of anti-periodic solutions to nonlinear parabolic equations in noncylindrical domains.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 14,
Issue. 9,
p.
771.
Okochi, Hiroko
1990.
A remark on the existence of periodic solutions to an evolution equation of parabolic-type.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 15,
Issue. 10,
p.
991.
Okochi, Hiroko
1992.
Asymptotic behavior of solutions to certain nonlinear parabolic evolution equations. II.
Hiroshima Mathematical Journal,
Vol. 22,
Issue. 2,
Ôtani, Mitsuharu
1995.
Almost periodic solutions of periodic systems governed by subdifferential operators.
Proceedings of the American Mathematical Society,
Vol. 123,
Issue. 6,
p.
1827.
an Ton, Bui
1996.
On an optimal control problem for a parabolic inclusion.
Nagoya Mathematical Journal,
Vol. 143,
Issue. ,
p.
195.
Lieberman, Gary M
2001.
Time-Periodic Solutions of Quasilinear Parabolic Differential Equations.
Journal of Mathematical Analysis and Applications,
Vol. 264,
Issue. 2,
p.
617.
SAAL, Jürgen
2006.
Maximal regularity for the Stokes system on noncylindrical space-time domains.
Journal of the Mathematical Society of Japan,
Vol. 58,
Issue. 3,
Hieber, Matthias
and
Saal, Jürgen
2016.
Handbook of Mathematical Analysis in Mechanics of Viscous Fluids.
p.
1.
Calvo, Juan
Novaga, Matteo
and
Orlandi, Giandomenico
2017.
Parabolic equations in time-dependent domains.
Journal of Evolution Equations,
Vol. 17,
Issue. 2,
p.
781.
S. Papageorgiou, Nikolaos
and
D. Rădulescu, Vicenţiu
2017.
Periodic solutions for time-dependent subdifferential evolution inclusions.
Evolution Equations & Control Theory,
Vol. 6,
Issue. 2,
p.
277.
Ôtani, Mitsuharu
and
Uchida, Shun
2018.
Existence of Time Periodic Solution to Some Double-Diffusive Convection System in the Whole Space Domain.
Journal of Mathematical Fluid Mechanics,
Vol. 20,
Issue. 3,
p.
1035.
Hieber, Matthias
and
Saal, Jürgen
2018.
Handbook of Mathematical Analysis in Mechanics of Viscous Fluids.
p.
117.
Bin, Chen
and
Timoshin, Sergey A.
2022.
Periodic Solutions of a Phase-Field Model with Hysteresis.
Applied Mathematics & Optimization,
Vol. 85,
Issue. 1,
Timoshin, Sergey A.
and
Wang, Yifu
2024.
Periodic solutions of a population dynamics model with hysteresis.
Nonlinear Analysis: Real World Applications,
Vol. 77,
Issue. ,
p.
104050.
Kubo, Masahiro
and
Yamazaki, Noriaki
2024.
Periodic solutions to a class of quasi-variational evolution equations.
Journal of Differential Equations,
Vol. 384,
Issue. ,
p.
165.