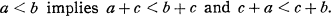Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Saitô, Tôru
1968.
The Archimedean property in an ordered semigroup.
Journal of the Australian Mathematical Society,
Vol. 8,
Issue. 3,
p.
547.
Weinert, Hanns Joachim
1969.
On the extension of partial orders on semigroups of right quotients..
Transactions of the American Mathematical Society,
Vol. 142,
Issue. 0,
p.
345.
Dauns, John
1981.
Algebra Carbondale 1980.
Vol. 848,
Issue. ,
p.
235.
1981.
Linear and Combinatorial Optimization in Ordered Algebraic Structures.
Vol. 10,
Issue. ,
p.
339.
Dauns, John
1982.
Generalized skew polynomial rings.
Transactions of the American Mathematical Society,
Vol. 271,
Issue. 2,
p.
575.
Brungs, Hans Heinrich
and
Törner, Günter
1987.
Right invariant right holoids.
Communications in Algebra,
Vol. 15,
Issue. 5,
p.
985.
Dauns, John
1989.
Centers of semigroup rings and conjugacy classes.
Semigroup Forum,
Vol. 38,
Issue. 1,
p.
355.
Brungs, H. H.
and
Törner, G.
1989.
A structure theorem for right invariant right holoids.
Semigroup Forum,
Vol. 39,
Issue. 1,
p.
39.
Davvaz, B.
Corsini, P.
and
Changphas, T.
2015.
Relationship between ordered semihypergroups and ordered semigroups by using pseudoorder.
European Journal of Combinatorics,
Vol. 44,
Issue. ,
p.
208.
Omidi, Saber
Davvaz, Bijan
and
Zhan, Jian Ming
2017.
An investigation on ordered algebraic hyperstructures.
Acta Mathematica Sinica, English Series,
Vol. 33,
Issue. 8,
p.
1107.
Pornsurat, Patchara
Palakawong na Ayutthaya, Pakorn
and
Pibaljommee, Bundit
2021.
Prime i-Ideals in Ordered n-ary Semigroups.
Mathematics,
Vol. 9,
Issue. 5,
p.
491.
Tang, Jian
and
Yaqoob, Naveed
2021.
A novel investigation on fuzzy hyperideals in ordered $$*$$-semihypergroups.
Computational and Applied Mathematics,
Vol. 40,
Issue. 2,