Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Manni, R. Salvati
1996.
On the differential of applications defined on moduli spaces of p.p.a.v. with level theta structure.
Mathematische Zeitschrift,
Vol. 221,
Issue. 1,
p.
231.
Salvati Manni, Riccardo
2006.
On the varieties defined by Riemann-Mumford’s relations.
Proceedings of the American Mathematical Society,
Vol. 135,
Issue. 5,
p.
1241.
Grushevsky, Samuel
and
Salvati Manni, Riccardo
2013.
The Scorza correspondence in genus 3.
Manuscripta Mathematica,
Vol. 141,
Issue. 1-2,
p.
111.


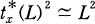 .
.