Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Oda, Tadao
1985.
Recent Progress of Algebraic Geometry in Japan.
Vol. 73,
Issue. ,
p.
188.
Müller, W.
Schmidt, M.
and
Schrader, R.
1998.
Hyperelliptic Riemann surfaces of infinite genus and
solutions of the KdV equation.
Duke Mathematical Journal,
Vol. 91,
Issue. 2,


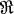 of infinite genus such that the Riemann’s theta functions associated with
of infinite genus such that the Riemann’s theta functions associated with 