Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Shirai, Susumu
1978.
A remark concerning the 2-adic number field.
Nagoya Mathematical Journal,
Vol. 71,
Issue. ,
p.
87.
Shirai, Susumu
1979.
On Galois groups of class two extensions over the rational number field.
Nagoya Mathematical Journal,
Vol. 75,
Issue. ,
p.
121.
Shirai, Susumu
1979.
On the central ideal class group of cyclotomic fields.
Nagoya Mathematical Journal,
Vol. 75,
Issue. ,
p.
133.
Furuta, Yoshiomi
1980.
A prime decomposition symbol for a non-abelian central extension which is abelian over a bicyclic biquadratic field.
Nagoya Mathematical Journal,
Vol. 79,
Issue. ,
p.
79.
Miyake, Katsuya
1983.
Central extensions and Schur’s multiplicators of Galois groups.
Nagoya Mathematical Journal,
Vol. 90,
Issue. ,
p.
137.
Opolka, Hans
1983.
Extensions of number fields defined by cohomology groups.
Nagoya Mathematical Journal,
Vol. 92,
Issue. ,
p.
179.
Heider, Franz-Peter
1984.
Kapitulationsproblem und knotentheorie.
Manuscripta Mathematica,
Vol. 46,
Issue. 1-3,
p.
229.
Miyake, Katsuya
1984.
On central extensions of a Galois extension of algebraic number fields.
Nagoya Mathematical Journal,
Vol. 93,
Issue. ,
p.
133.
Kuz'min, L. V.
1987.
Algebraic number fields.
Journal of Soviet Mathematics,
Vol. 38,
Issue. 3,
p.
1930.
TAKEUCHI, Teruo
1994.
The Hasse norm principle modulo <i>m</i> of finite Galois extensions of algebraic number fields and a generalization of a theorem of Fröhlich on the <i>e</i>-divisibility of the class numbers of <i>e</i>-abelian fields.
Japanese journal of mathematics. New series,
Vol. 20,
Issue. 2,
p.
231.
Yamashita, Hiroshi
1999.
On the rank of the first radical layer of ap-class group of an algebraic number field.
Nagoya Mathematical Journal,
Vol. 156,
Issue. ,
p.
85.


 of Galois extensions of an algebraic number field
of Galois extensions of an algebraic number field whose degree is some power of a prime
whose degree is some power of a prime 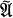 be the module of
be the module of 